Advertisements
Advertisements
Question
In each of the figures [(i)-(iv)] given below, a line segment is drawn parallel to one side of the triangle and the lengths of certain line-segment are marked. Find the value of x in each of the following :
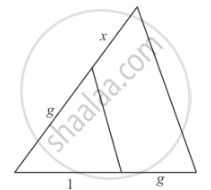
Solution
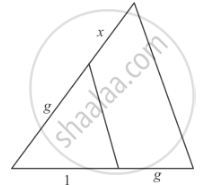
`x/(x+g)=g/(g+1)`
By cross multiplication on both sides, we get
`x xx(g+1)=gxx(x+g)`
`xg+x=gx+g^2`
`x= gx +g^2-gx`
`x= cancel(gx)+g^2-cancel(gx)`
`x= g^2`
Hence the value of x is `g^2`.
APPEARS IN
RELATED QUESTIONS
In below figure, If AB || CD, find the value of x.

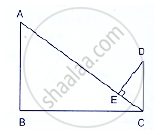
In Figure below, if AB ⊥ BC, DC ⊥ BC and DE ⊥ AC, Prove that Δ CED ~ ABC.
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AD = 5.7cm, DB = 9.5cm, AE = 4.8cm and EC = 8cm.

In the given figure, DE || BD. Determine AC and AE.

ABCD is a trapezium having AB || DC. Prove that O, the point of intersection of diagonals, divides the two diagonals in the same ratio. Also prove that
In ∆ABC, if BD ⊥ AC and BC2 = 2 AC . CD, then prove that AB = AC.
In ∆ABC, given that AB = AC and BD ⊥ AC. Prove that BC2 = 2 AC. CD
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio.
If ∆ABC and ∆DEF are similar such that 2AB = DE and BC = 8 cm, then EF =
In a ∆ABC, ∠A = 90°, AB = 5 cm and AC = 12 cm. If AD ⊥ BC, then AD =
