Advertisements
Advertisements
Question
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sin theta = sqrt3/2`
Solution
`sin theta = sqrt3/2`
We know `sin theta = "opposide side"/"Hyotence" = sqrt3/2`
Now consider right-angled Δle ABC
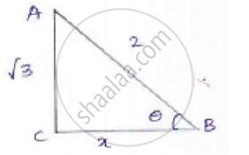
Let x = adjacent sidead
By applying Pythagoras
𝐴𝐵2 = 𝐴𝐶2 + 𝐵𝐶2
4 = 3+𝑥2
𝑥2 = 4 − 3
𝑥2 = 1
𝑥 = 1
`cos = "opposite side"/"Hypotenuse" = 1/2`
`tan = "Oppsite side"/"hypotenuse" = sqrt3/1 = sqrt3`
`cosec theta = 1/sin theta = 1/(sqrt3/2) = 2/sqrt3`
sec = `1/cos theta = (1/1)/2 = 2`
`cot = 1/tan theta = 1/sqrt3`
APPEARS IN
RELATED QUESTIONS
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`cos A = 4/5`
If 3 cot θ = 2, find the value of `(4sin theta - 3 cos theta)/(2 sin theta + 6cos theta)`.
If `cos theta = 12/13`, show that `sin theta (1 - tan theta) = 35/156`
If `cot theta = 1/sqrt3` show that `(1 - cos^2 theta)/(2 - sin^2 theta) = 3/5`
If `tan theta = 1/sqrt7` `(cosec^2 theta - sec^2 theta)/(cosec^2 theta + sec^2 theta) = 3/4`
if `sin theta = 3/4` prove that `sqrt(cosec^2 theta - cot)/(sec^2 theta - 1) = sqrt7/3`
If cos A = `4/5`, then the value of tan A is ______.
If f(x) = `3cos(x + (5π)/6) - 5sinx + 2`, then maximum value of f(x) is ______.
If b = `(3 + cot π/8 + cot (11π)/24 - cot (5π)/24)`, then the value of `|bsqrt(2)|` is ______.
In ΔBC, right angled at C, if tan A = `8/7`, then the value of cot B is ______.