Advertisements
Advertisements
Question
In the figure, given below, AB = AC.
Prove that: ∠BOC = ∠ACD.

Solution

Let ∠ABO = ∠OBC = x and ∠ACO = ∠OCB = y
In ΔABC,
∠BAC = 180° - 2x - 2y ...(i)
Since, ∠B = ∠C ...[AB = AC]
`1/2"B" = 1/2"C"`
⇒ x = y
Now,
∠ACD = 2x + ∠BAC ...[Exterior angle is equal to sum of opp. interior angles]
∠ACD = 2x + 180° - 2x - 2y ...[From (i)]
∠ACD = 180° - 2y ...(i)
In ΔOBC,
∠BOC = 180° - x - y
⇒ ∠BOC = 180° - y - y ...[Already proved]
⇒ ∠BOC = 180° - 2y ...(ii)
From (i) and (ii)
∠BOC = ∠ACD
APPEARS IN
RELATED QUESTIONS
Calculate x :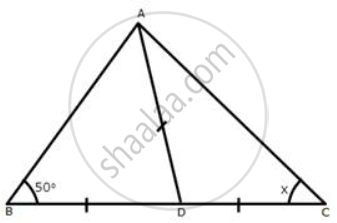
In the given figure; AB = BC and AD = EC.
Prove that: BD = BE.
Prove that a triangle ABC is isosceles, if: altitude AD bisects angles BAC.
Prove that a triangle ABC is isosceles, if: bisector of angle BAC is perpendicular to base BC.
Using the information given of the following figure, find the values of a and b.

If the equal sides of an isosceles triangle are produced, prove that the exterior angles so formed are obtuse and equal.
In isosceles triangle ABC, AB = AC. The side BA is produced to D such that BA = AD.
Prove that: ∠BCD = 90°
ABC is a triangle. The bisector of the angle BCA meets AB in X. A point Y lies on CX such that AX = AY.
Prove that: ∠CAY = ∠ABC.
Use the given figure to prove that, AB = AC.
The bisectors of the equal angles B and C of an isosceles triangle ABC meet at O. Prove that AO bisects angle A.
