Advertisements
Advertisements
Question
In the given figure; AB = BC and AD = EC.
Prove that: BD = BE.
Solution
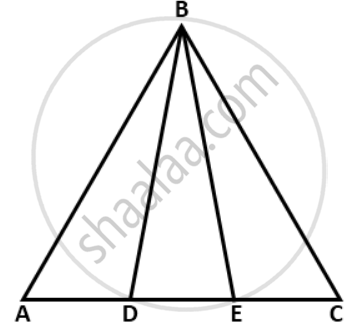
In ΔABC,
AB = BC .......(given)
⇒ ∠BCA = ∠BAC .......(Angles opposite to equal sides are equal)
⇒ ∠BCD = ∠BAE ….(i)
Given, AD = EC
⇒ AD + DE = EC + DE ...(Adding DE on both sides)
⇒ AE = CD .....….(ii)
Now, in triangles ABE and CBD,
AB = BC .....(given)
∠BAE = ∠BCD ....[From (i)]
AE = CD ......[From (ii)]
⇒ ΔABE ≅ ΔCBD
⇒ BE = BD ....(cpct)
APPEARS IN
RELATED QUESTIONS
An isosceles triangle ABC has AC = BC. CD bisects AB at D and ∠CAB = 55°.
Find:
- ∠DCB
- ∠CBD
In the figure given below, LM = LN; angle PLN = 110o.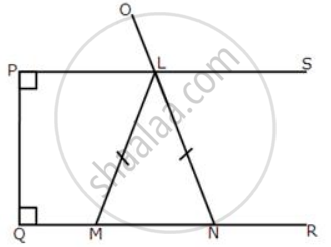
calculate: (i) ∠LMN
(ii) ∠MLN
In the figure, given below, AB = AC.
Prove that: ∠BOC = ∠ACD.

Calculate x :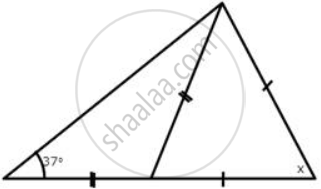
Prove that a triangle ABC is isosceles, if: altitude AD bisects angles BAC.
Prove that a triangle ABC is isosceles, if: bisector of angle BAC is perpendicular to base BC.
In triangle ABC; AB = AC and ∠A : ∠B = 8 : 5; find angle A.
Using the information given of the following figure, find the values of a and b.

Prove that the medians corresponding to equal sides of an isosceles triangle are equal.
The bisectors of the equal angles B and C of an isosceles triangle ABC meet at O. Prove that AO bisects angle A.
