Advertisements
Advertisements
Question
If the equal sides of an isosceles triangle are produced, prove that the exterior angles so formed are obtuse and equal.
Solution

Const: AB is produced to D and AC is produced to E so that exterior angles ∠DBC and ∠ECB are formed.
In ΔABC,
AB = AC ........[ Given ]
∴ ∠C = ∠B .....(i) [angels opp. to equal sides are equal]
Since angle B and angle C are acute they cannot be right angles or obtuse angles.
∠ABC + ∠DBC =180° .......[ABD is a st. line]
∠DBC = 180° − ∠ABC
∠DBC = 180° − ∠B ......(ii)
Similarly,
∠ACB + ∠ECB = 180° .......[ABD is a st. line]
∠ECB = 180° − ∠ACB
∠ECB = 180° − ∠C ........(iii)
∠ECB = 180° − ∠B .......(iv) [from(i) and (iii)]
∠DBC = ∠ECB ........[from (ii) and (iv)]
Now,
∠DBC = 180° − ∠B
But ∠B = Acute angel
∴ ∠DBC = 180° − Acute angle = obtuse angle
Similarly,
∠ECB = 180° − ∠C.
But ∠C = Acute angel
∴ ∠ECB = 180° − Acute angle = obtuse angle
Therefore, exterior angles formed are obtuse and equal.
APPEARS IN
RELATED QUESTIONS
Calculate x :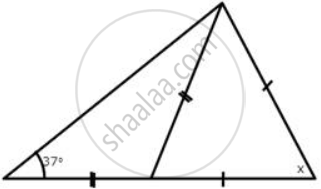
Calculate x :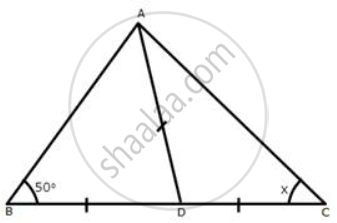
Prove that a triangle ABC is isosceles, if: altitude AD bisects angles BAC.
Prove that a triangle ABC is isosceles, if: bisector of angle BAC is perpendicular to base BC.
In the given figure, AD = AB = AC, BD is parallel to CA and angle ACB = 65°. Find angle DAC.
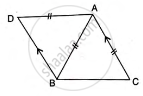
Using the information given of the following figure, find the values of a and b.

In isosceles triangle ABC, AB = AC. The side BA is produced to D such that BA = AD.
Prove that: ∠BCD = 90°
ABC is a triangle. The bisector of the angle BCA meets AB in X. A point Y lies on CX such that AX = AY.
Prove that: ∠CAY = ∠ABC.
Use the given figure to prove that, AB = AC.
Prove that the medians corresponding to equal sides of an isosceles triangle are equal.
