Advertisements
Advertisements
Question
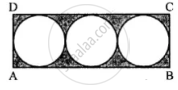
In the following figure, a rectangle ABCD enclosed three circles. If BC = 14 cm, find the area of the shaded portion (Take π = 22/7)
Advertisements
Solution
AB = 14 + 14 + 14 + 7
= 49 cm
BC = 14 cm
AB = 49 cm
Area of ABCD = 49 × 14 cm2
Diameter of circle = 14 cm
Radius = `"Diameter"/2 = 7 cm`
Area of the circle = πr2
Area of the circle = `22/7xx7^2`
= `22/7xx49`
= `1078/7`
= 154 cm2
Area of 3 circles = 3 × 154 cm2
= 462 cm2
Area of the semicircle = `1/2` πr2
= `1/2xx22/7xx7^2`
= `22/14xx49`
= `1078/14`
= 77 cm2
⇒ Area of shaded portion = 686 – (462 + 77) cm2
= 686 – 539 cm2
= 147 cm2
Hence, the area of the shaded portion is 147 cm2.
APPEARS IN
RELATED QUESTIONS
From a circular card sheet of radius 14 cm, two circles of radius 3.5 cm and a rectangle of length 3 cm and breadth 1 cm are removed. (as shown in the following figure). Find the area of the remaining sheet. (Take `pi = 22/7`)
Find the area of a parallelogram with base equal to 25 cm and the corresponding height measuring 16.8 cm.
From each of the two opposite corners of a square of side 8 cm, a quadrant of a circle of radius 1.4 cm is cut. Another circle of radius 4.2 cm is also cut from the centre as shown in the following figure. Find the area of the remaining (Shaded) portion of the square. (Use π = 22/7)
In the following figure, OABC is a square of side 7 cm. If OAPC is a quadrant of a circle with centre O, then find the area of the shaded region. (Use π = 22/7)
In the the following figure, PSR, RTQ and PAQ are three semicircles of diameter 10 cm, 3 cm and 7 cm respectively. Find the perimeter of shaded region.
In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the area of the cross-section.
What is the area of a sector of a circle of radius 5 cm formed by an arc of length 3.5 cm?
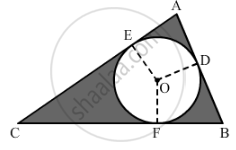
In the given figure, ∆ABC is right-angled at A. Find the area of the shaded region if AB = 6 cm, BC = 10 cm and O is the centre of the incircle of ∆ABC.
A square tank has an area of 1600 cm2. There are four semicircular plots around it. Find the cost of turfing the plots at Rs 12.50 per m2
A square is inscribed in a circle of radius 6 cm. Find the area of the square. Give your answer correct to two decimal places if `sqrt(2)` = 1.414.
