Advertisements
Advertisements
Question
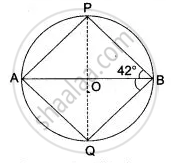
In the following figure, O is the centre of the circle, ∠ PBA = 42°.
Calculate:
(i) ∠ APB
(ii) ∠PQB
(iii) ∠ AQB
Solution
(i) In circle C(O, r)
AB is the diameter.
So, ∠ APB = 90° ....(Angle in a semicircle)
(ii) Now in Δ APB,
∠ PAB = 180° - (∠ APB + ∠ ABP)
∠ PAB = 180° - ( 90° + 42°)
∠ PAB = 180° - 132°
∠ PAB = 48°
∠ PQB = ∠ PAB = 48° ....(Angle of the same segment)
Hence,
∠ PQB = 48°
(iii) AQBP is a cyclic quadrilateral.
∠ APB + ∠ AQB = 180°
⇒ 90° + ∠ AQB = 180°
⇒ ∠ AQB = 180° - 90° = 90°.
APPEARS IN
RELATED QUESTIONS
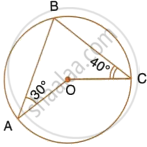
In the given figure, O is the centre of the circle. ∠OAB and ∠OCB are 30° and 40° respectively. Find ∠AOC . Show your steps of working.
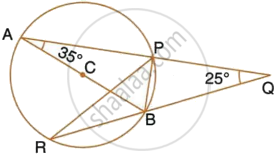
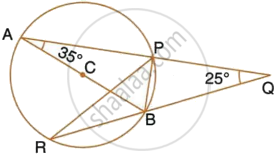
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find : ∠PRB
A triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that:
- ∠ABC = 2∠APQ,
- ∠ACB = 2∠APR,
- `∠QPR = 90^circ - 1/2 ∠BAC`.
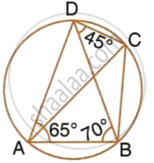
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find:
- ∠BCD
- ∠ACB
Hence, show that AC is a diameter.
In a cyclic-trapezium, the non-parallel sides are equal and the diagonals are also equal. Prove it.
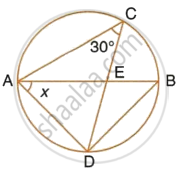
In the given circle with diameter AB, find the value of x.
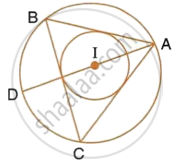
If I is the incentre of triangle ABC and AI when produced meets the circumcircle of triangle ABC in point D. If ∠BAC = 66° and ∠ABC = 80°.
Calculate:
- ∠DBC,
- ∠IBC,
- ∠BIC.
In cyclic quadrilateral ABCD; AD = BC, ∠BAC = 30° and ∠CBD = 70°; find:
- ∠BCD
- ∠BCA
- ∠ABC
- ∠ADC
AB is a diameter of the circle APBR, as shown in the figure. APQ and RBQ are straight lines. Find : ∠BPR
If I is the incentre of triangle ABC and AI when produced meets the cicrumcircle of triangle ABC in points D . if ∠BAC = 66° and ∠ABC = 80°. Calculate : ∠IBC