Advertisements
Advertisements
Question
In the given figure ABC and CEF are two triangles where BA is parallel to CE and AF: AC = 5: 8.
(i) Prove that ΔADF ∼ ΔCEF
(ii) Find AD if CE = 6 cm
(iii) If DF is parallel to BC find area of ΔADF: area of ΔABC.
Solution
(i) In ΔADF and ΔCFE
∠DAF = ∠FCE ...(alternate angles)
∠AFD = ∠CEF ...(vertically opp. angles)
∠ADF = ∠CEF
∴ ΔADF ∼ ΔCEF ...(by A.A.)
Hence Proved.
(ii) ΔADF ∼ ΔCEF
∴ `"AD"/"CE" = "AF"/"FC"`
FC = AC - AF
= 8 - 5 = 3
∴ `"AD"/(6) = (5)/(3)`
⇒ AD = 10 cm.
(iii) DF | | BC ∴ ΔADF ∼ ΔABC
∵ ∠D = ∠B and ∠F = ∠C.
∴ `"Ar. of ΔADF"/"Ar. of ΔABC" = "AF"^2/"AC"^2`
= `(5/8)^2 = (25)/(64)`.
APPEARS IN
RELATED QUESTIONS
In a triangle ABC, line l || Side BC and line l intersects side AB and AC in points P and Q, respectively. Prove that: `"AP"/"BP"="AQ"/"QC"`

Given `triangle ABC ~ triangle PQR`, if `(AB)/(PQ) = 1/3`, then find `(ar triangle ABC)/(ar triangle PQR)`
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm. L is a point on PR such that RL : LP = 2 : 3. QL produced meets RS at M and PS produced at N.

Find the lengths of PN and RM.
P and Q are points on the sides AB and AC respectively of a ΔABC. If AP = 2cm, PB = 4cm, AQ = 3cm and QC = 6cm, show that BC = 3PQ.
In ΔABC, D and E are the mid-point on AB and AC such that DE || BC.
If AD = 4x - 3, AE = 8x - 7, BD = 3x - 1 and CE = 5x - 3,Find x.
ΔABC has been reduced by a scale factor 0.6 to ΔA'B'C'/ Calculate:Length of B' C', if BC = 8cm
On a map drawn to a scale of 1:25000, a rectangular plot of land has sides 12cm x 16cm. Calculate: The area of the plot in sq km
A girl looks the reflection of the top of the lamp post on the mirror which is 6.6 m away from the foot of the lamppost. The girl whose height is 1.25 m is standing 2.5 m away from the mirror. Assuming the mirror is placed on the ground facing the sky and the girl, mirror and the lamppost are in the same line, find the height of the lamp post.
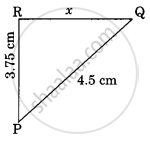
In the given figure ΔABC ~ ΔPQR. The value of x is
 |
 |
In figure, if AD = 6 cm, DB = 9 cm, AE = 8 cm and EC = 12 cm and ∠ADE = 48°. Find ∠ABC.

