Advertisements
Advertisements
Question
In the given figure, diagonals PR and QS of the parallelogram PQRS intersect at point O and LM is parallel to PS. Show that:
(i) 2 Area (POS) = Area (// gm PMLS)
(ii) Area (POS) + Area (QOR) = Area (// gm PQRS)
(iii) Area (POS) + Area (QOR) = Area (POQ) + Area (SOR).
Solution
(i) Since POS and parallelogram, PMLS are on the same base PS and between the same parallels i.e. SP//LM.
As O is the center of LM and the Ratio of the area of triangles with the same vertex and bases along the same line is equal to the ratio of their respective bases.
The area of the parallelogram is twice the area of the triangle if they lie on the same base and in between the same parallels.
So 2(Area of PSO)=Area of PMLS
Hence Proved.
(ii) Consider the expression: Area ( ΔPOS) + Area ( QOR ):
LM is parallel to PS and PS is parallel to RQ, therefore, LM is
Since triangle POS lie on the base PS and in between the parallels PS and LM, we have,
Area ( ΔPOS ) = `1/2"Area"( square` PSLM )
Since triangle QOR lie on the base QR and in between the Parallels LM and RQ, we have,
Area ( ΔQOR ) = `1/2"Area" ( square` LMQR )
Area ( ΔPOS ) + Area ( ΔQOR ) = `1/2"Area"( square` PSLM ) + `1/2"Area"( square` LMQR )
= `1/2 ["Area (PSLM )" + "Area" ( square` LMQR )]
= `1/2["Area" ( square` PQRS) ]
(iii) In a parallelogram, the diagonals bisect each other.
Therefore, OS = OQ
Consider the triangle PQS, since OS = OQ, OP is the median of the triangle PQS.
We know that the median of a triangle divides it into two triangles of equal area.
Therefore,
Area ( ΔPOS) = Area ( ΔPOQ ) ....(1)
Similarly, since OR is the median of the triangle QRS, we have, Area ( ΔQOR ) = Area ( ΔSOR ) ....(2)
Adding equations (1) and (2), we have,
Area ( ΔPOS ) + Area( ΔQOR) = Area ( ΔPOQ ) + Area( SOR)
Hence Proved.
APPEARS IN
RELATED QUESTIONS
In the given figure, AD // BE // CF.
Prove that area (ΔAEC) = area (ΔDBF)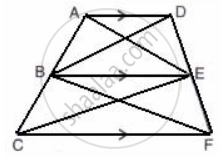
ABCD is a trapezium with AB // DC. A line parallel to AC intersects AB at point M and BC at point N.
Prove that: area of Δ ADM = area of Δ ACN.
In the given figure, D is mid-point of side AB of ΔABC and BDEC is a parallelogram.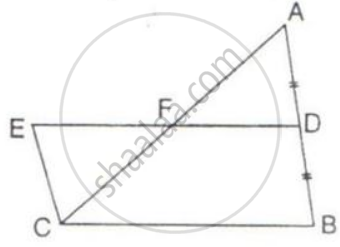
Prove that: Area of ABC = Area of // gm BDEC.
ABCD and BCFE are parallelograms. If area of triangle EBC = 480 cm2; AB = 30 cm and BC = 40 cm.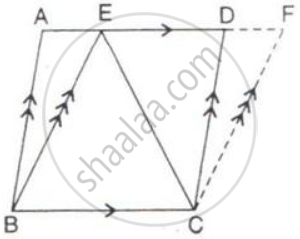
Calculate :
(i) Area of parallelogram ABCD;
(ii) Area of the parallelogram BCFE;
(iii) Length of altitude from A on CD;
(iv) Area of triangle ECF.
In the following figure, DE is parallel to BC.
Show that:
(i) Area ( ΔADC ) = Area( ΔAEB ).
(ii) Area ( ΔBOD ) = Area( ΔCOE ).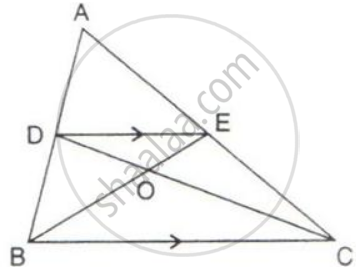
In the given figure, AP is parallel to BC, BP is parallel to CQ.
Prove that the area of triangles ABC and BQP are equal.
ABCD is a parallelogram in which BC is produced to E such that CE = BC and AE intersects CD at F.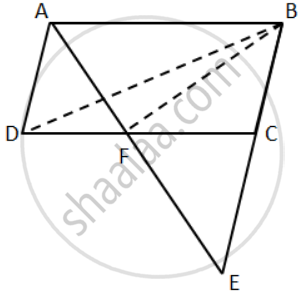
If ar.(∆DFB) = 30 cm2; find the area of parallelogram.
In the following figure, BD is parallel to CA, E is mid-point of CA and BD = `1/2`CA
Prove that: ar. ( ΔABC ) = 2 x ar.( ΔDBC )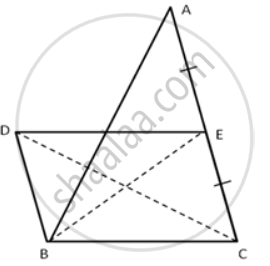
In ΔABC, E and F are mid-points of sides AB and AC respectively. If BF and CE intersect each other at point O,
prove that the ΔOBC and quadrilateral AEOF are equal in area.
In parallelogram ABCD, P is the mid-point of AB. CP and BD intersect each other at point O. If the area of ΔPOB = 40 cm2, and OP: OC = 1:2, find:
(i) Areas of ΔBOC and ΔPBC
(ii) Areas of ΔABC and parallelogram ABCD.
