Advertisements
Advertisements
Question
In the given figure, PQ = PS. The value of x is ______.

Options
35°
45°
55°
70°
Solution
In the given figure, PQ = PS. The value of x is 45°.
Explanation:
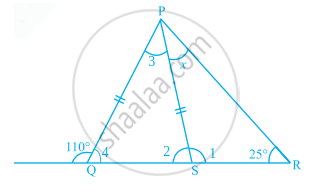
In ΔPQS, ∠2 + ∠3 = 110° ......(i) [Exterior angle property of a triangle]
∠2 + ∠3 + ∠4 = 180° ......[Angle sum property of a triangle]
⇒ ∠4 = 180° = 110° ......[Using (i)]
∴ ∠4 = 70°
Now, PQ = PS ......(Given)
⇒ ∠2 = ∠4 = 70° ......(ii)
(ii) Now, in ∆PRS,
∠2 = x + 25° ......[Exterior angle property of a triangle]
⇒ x = 70° – 25° = 45° ......[Using (ii)]
APPEARS IN
RELATED QUESTIONS
Find the value of the unknown x in the following diagram:

Find the value of the unknown x in the following diagram:

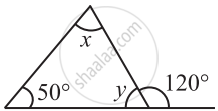
Find the value of the unknown x and y in the following diagram:
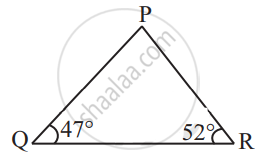
In the given figure find m∠P.
Observe the figure and find the value of ∠A + ∠N + ∠G + ∠L + ∠E + ∠S
In the given figure, which of the following statement is true?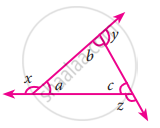
The angles of a triangle are in the ratio 5 : 3 : 7. The triangle is ______.
In the following figure, DE || QR and AP and BP are bisectors of ∠EAB and ∠RBA, respectively. Find ∠APB.

The angles of a triangle are in the ratio 2 : 3 : 4. Find the angles of the triangle.
In a right-angled triangle if an angle measures 35°, then find the measure of the third angle.
