Advertisements
Advertisements
Question
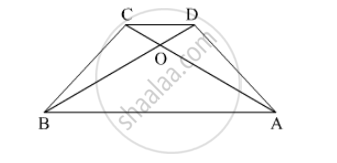
In trapezium ABCD, side AB || side DC, diagonals AC and BD intersect in point O. If AB = 20, DC = 6, OB = 15 then Find OD.
Solution
Given:
side AB || side DC
AB = 20,
DC = 6,
OB = 15
In △COD and △AOB
∠COD = ∠AOB (Vertically opposite angles)
∠CDO= ∠ABO (Alternate angles, CD || BA and BD is a transversal line)
By AA test of similarity
△COD ∼ △AOB
∴ `(CD)/(AB) = (OD)/(OB)` (Corresponding sides are proportional)
\[ \Rightarrow \frac{6}{20} = \frac{OD}{15}\]
\[ \Rightarrow OD = 4 . 5\]
APPEARS IN
RELATED QUESTIONS
The ratio of the areas of two triangles with the common base is 14 : 9. Height of the larger triangle is 7 cm, then find the corresponding height of the smaller triangle.
In the following figure RP: PK= 3:2, then find the value of A(ΔTRP):A(ΔTPK).

In the given figure, BC ⊥ AB, AD ⊥ AB, BC = 4, AD = 8, then find `("A"(∆"ABC"))/("A"(∆"ADB"))`
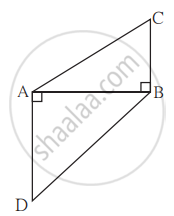
In adjoining figure, PQ ⊥ BC, AD ⊥ BC then find following ratios.

- `("A"(∆"PQB"))/("A"(∆"PBC"))`
- `("A"(∆"PBC"))/("A"(∆"ABC"))`
- `("A"(∆"ABC"))/("A"(∆"ADC"))`
- `("A"(∆"ADC"))/("A"(∆"PQC"))`
In trapezium PQRS, side PQ || side SR, AR = 5AP, AS = 5AQ then prove that, SR = 5PQ
In ∆ABC, B - D - C and BD = 7, BC = 20 then find following ratio.
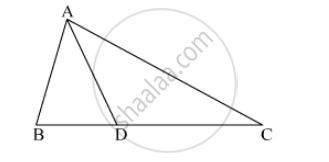
`"A(∆ ABD)"/"A(∆ ADC)"`
In ∆ABC, B – D – C and BD = 7, BC = 20, then find the following ratio.
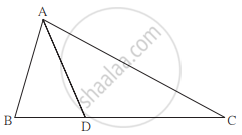
`(A(∆ABD))/(A(∆ABC))`
In ∆ABC, B – D – C and BD = 7, BC = 20 then Find following ratio.

\[\frac{A\left( ∆ ADC \right)}{A\left( ∆ ABC \right)}\]
In the given, seg BE ⊥ seg AB and seg BA ⊥ seg AD.
if BE = 6 and AD = 9 find `(A(Δ ABE))/(A(Δ BAD))`.

If ΔXYZ ~ ΔPQR then `"XY"/"PQ" = "YZ"/"QR"` = ?
Areas of two similar triangles are in the ratio 144: 49. Find the ratio of their corresponding sides.
In fig., TP = 10 cm, PS = 6 cm. `"A(ΔRTP)"/"A(ΔRPS)"` = ?
In fig., PM = 10 cm, A(ΔPQS) = 100 sq.cm, A(ΔQRS) = 110 sq.cm, then NR?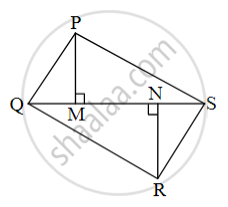
ΔPQS and ΔQRS having seg QS common base.
Areas of two triangles whose base is common are in proportion of their corresponding [______]
`("A"("PQS"))/("A"("QRS")) = (["______"])/"NR"`,
`100/110 = (["______"])/"NR"`,
NR = [ ______ ] cm
From adjoining figure, ∠ABC = 90°, ∠DCB = 90°, AB = 6, DC = 8, then `("A"(Δ"ABC"))/("A"(Δ"BCD"))` = ?

Prove that, The areas of two triangles with the same height are in proportion to their corresponding bases. To prove this theorem start as follows:
- Draw two triangles, give the names of all points, and show heights.
- Write 'Given' and 'To prove' from the figure drawn.
If ΔABC ∼ ΔDEF, length of side AB is 9 cm and length of side DE is 12 cm, then find the ratio of their corresponding areas.
