Advertisements
Advertisements
Question
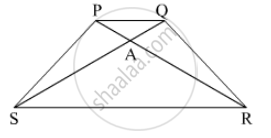
In trapezium PQRS, side PQ || side SR, AR = 5AP, AS = 5AQ then prove that, SR = 5PQ
Solution
Given:
side PQ || side SR
AR = 5AP,
AS = 5AQ
To prove: SR = 5PQ
Proof: In ∆APQ and ∆ARS
∠PAQ = ∠RAS (Vertically Opposite angles)
∠PQA = ∠RSA (Alternate angles, side PQ || side SR and QS is a transversal line)
By AA test of similarity
∆APQ ~ ∆ARS
RELATED QUESTIONS
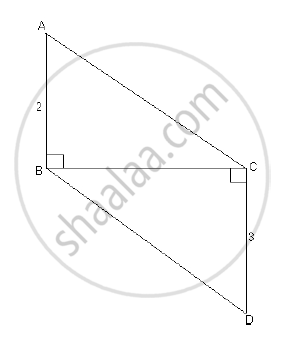
In the following figure seg AB ⊥ seg BC, seg DC ⊥ seg BC. If AB = 2 and DC = 3, find
The ratio of the areas of two triangles with the common base is 14 : 9. Height of the larger triangle is 7 cm, then find the corresponding height of the smaller triangle.
In adjoining figure, PQ ⊥ BC, AD ⊥ BC then find following ratios.

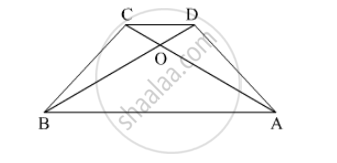
In trapezium ABCD, side AB || side DC, diagonals AC and BD intersect in point O. If AB = 20, DC = 6, OB = 15 then Find OD.
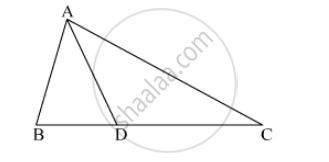
In ∆ABC, B - D - C and BD = 7, BC = 20 then find following ratio.
Ratio of areas of two triangles with equal heights is 2 : 3. If base of the smaller triangle is 6 cm then what is the corresponding base of the bigger triangle ?
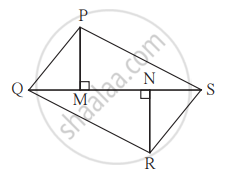
In the figure, PM = 10 cm, A(∆PQS) = 100 sq.cm, A(∆QRS) = 110 sq. cm, then find NR.
The ratio of the areas of two triangles with the common base is 4 : 3. Height of the larger triangle is 2 cm, then find the corresponding height of the smaller triangle.
In ∆ABC, B – D – C and BD = 7, BC = 20, then find the following ratio.
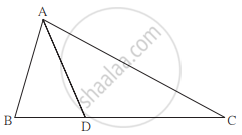
In ∆ABC, B – D – C and BD = 7, BC = 20 then Find following ratio.

If ΔXYZ ~ ΔPQR then
Areas of two similar triangles are in the ratio 144: 49. Find the ratio of their corresponding sides.
Ratio of corresponding sides of two similar triangles is 4:7, then find the ratio of their areas = ?
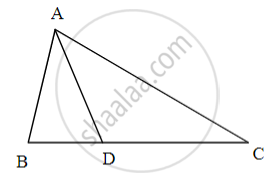
In fig. BD = 8, BC = 12, B-D-C, then 
In ΔABC, B − D − C and BD = 7, BC = 20, then find the following ratio.
(i)
(ii)
(iii)
Prove that, The areas of two triangles with the same height are in proportion to their corresponding bases. To prove this theorem start as follows:
- Draw two triangles, give the names of all points, and show heights.
- Write 'Given' and 'To prove' from the figure drawn.
If ΔABC ∼ ΔDEF, length of side AB is 9 cm and length of side DE is 12 cm, then find the ratio of their corresponding areas.
In the figure, PQ ⊥ BC, AD ⊥ BC. To find the ratio of A(ΔPQB) and A(ΔPBC), complete the following activity.
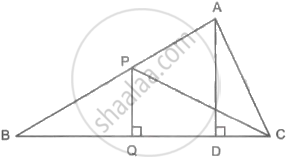
Given: PQ ⊥ BC, AD ⊥ BC
Now, A(ΔPQB) =
A(ΔPBC) =
Therefore,
=
