Advertisements
Advertisements
Question
In triangle PQR, angle Q = 90°, find: PQ, if PR = 34 cm and QR = 30 cm
Solution
Given:
PR = 34 cm
QR = 30 cm
PQ =?
∠PQR = 90°
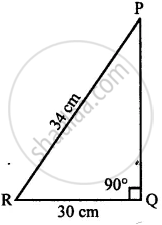
According to Pythagoras Theorem,
(PR)2 = (PQ)2 + (QR)2
(34)2 = PQ2 + (30)2
1156 = PQ2 + 900
1156 − 900 = PQ2
256 = PQ2
∴ PQ = `sqrt256` = 16 cm
APPEARS IN
RELATED QUESTIONS
ABC is an isosceles triangle with AC = BC. If AB2 = 2AC2, prove that ABC is a right triangle.
A 15 m long ladder reached a window 12 m high from the ground on placing it against a wall at a distance a. Find the distance of the foot of the ladder from the wall.

A tree is broken at a height of 5 m from the ground and its top touches the ground at a distance of 12 m from the base of the tree. Find the original height of the tree.
In the following figure, OP, OQ, and OR are drawn perpendiculars to the sides BC, CA and AB respectively of triangle ABC.
Prove that: AR2 + BP2 + CQ2 = AQ2 + CP2 + BR2

In the given figure, BL and CM are medians of a ∆ABC right-angled at A. Prove that 4 (BL2 + CM2) = 5 BC2.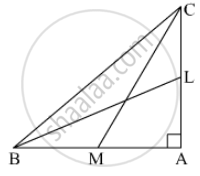
Triangle XYZ is right-angled at vertex Z. Calculate the length of YZ, if XY = 13 cm and XZ = 12 cm.
Find the Pythagorean triplet from among the following set of numbers.
4, 5, 6
In a right angled triangle, if length of hypotenuse is 25 cm and height is 7 cm, then what is the length of its base?
In the given figure, ∠T and ∠B are right angles. If the length of AT, BC and AS (in centimeters) are 15, 16, and 17 respectively, then the length of TC (in centimeters) is ______.

Two poles of 10 m and 15 m stand upright on a plane ground. If the distance between the tops is 13 m, find the distance between their feet.
