Advertisements
Advertisements
Question
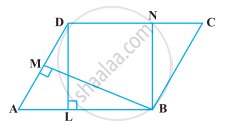
Medians of ΔABC intersect at G. If ar (ΔABC) = 27 cm2, then ar (ΔBGC) =
Options
6 cm2
9 cm2
12 cm2
18 cm2
Solution
Given: (1) Median of ΔABC meet at G.
(2) Area of ΔABC = 27 cm2
To find: Area of ΔBCG.
We know that the medians of the triangle divides each other in the ratio of 2:1

Hence,
ar (ΔBED) = `1/2 xx BC xx GD`
ar (ΔBED) =`1/2 xx BC xx 1/3 (AD)`
ar (ΔBED) =`1/3(1/2xx AD xx BC)`
ar (ΔBED) =`1/3 (Δ ABC)`
ar (ΔBED) = = `1/3(27)`
ar (ΔBED) == 9 cm2
APPEARS IN
RELATED QUESTIONS
In the given figure, ABCD is a rectangle in which CD = 6 cm, AD = 8 cm. Find the area of parallelogram CDEF.

In the given figure, ABCD is a rectangle with sides AB = 10 cm and AD = 5 cm. Find the area of ΔEFG.

The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm is ______.
In the given figure, PQRS is a parallelogram. If X and Y are mid-points of PQ and SRrespectively and diagonal Q is joined. The ratio ar (||gm XQRY) : ar (ΔQSR) =

Who had the bigger piece? How much bigger?
How many tiles like the triangle given here will fit in the white design?
Area of design = ________ square cm

If each square on this page is equal to 1 square meter of land, how much land will each of her children get? ________ square m
Each line gives a story. You have to choose the question which makes the best story problem. The first one is already marked.
- A shopkeeper has 204 eggs. He puts them in egg trays. Each tray has 12 eggs.
a) How many more eggs will he need? b) How many fresh eggs does he sell? c) How many egg trays does he need?
In the following figure, the area of parallelogram ABCD is ______.
Find the area of the following figure by counting squares: