Advertisements
Advertisements
Question
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
- What do you call the point O?
- What is the relation between the distances OA, OB and OC?
- Does the perpendicular bisector of BC pass through O?
Solution
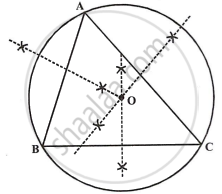
- O is called the circumcentre of circumcircle of ΔABC.
- OA, OB and OC are the radii of the circumcircle.
- Yes, the perpendicular bisector of BC will pass through O.
APPEARS IN
RELATED QUESTIONS
Construct a regular hexagon of side 5 cm. Hence construct all its lines of symmetry and name them.
Using ruler and compasses only,
- Construct a triangle ABC with the following data :
Base AB = 6 cm, BC = 6.2 cm and ∠CAB = 60°. - In the same diagram, draw a circle which passes through the points A, B and C and mark its center O.
- Draw a perpendicular from O to AB which meets AB in D.
- Prove that : AD = BD.
Using ruler and compasses only, construct and equilateral triangle with side 4.5 cm. Draw a circumcircle of this triangle and measure its radius.
Draw a circle with radius 3 cm and inscribe an equilateral triangle in it.
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
What is the relation between the distances OA, OB and OC?
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
Does the perpendicular bisector of BC pass through O?
Construct a triangle ABC, given that the radius of the circumcircle of triangle ABC is 3.5 cm, ∠ BCA = 45° and ∠ BAC = 60°.
Using ruler and compass only, construct a triangle ABC such that AB = 5 cm, ABC = 75°, and the radius of the circumcircle of triangle ABC is 3.5 cm. On the same diagram, construct a circle, touching AB at its middle point and also touching the side AC.
Use ruler and compasses only for this question:
(i) Construct A ABC, where AB = 3.5 cm, BC = 6 cm and ∠ ABC = 60°.
(ii) Construct the locus of points inside the triangle which are equidistant from BA and BC.
(iii) Construct the locus of points inside the triangle which are equidistant from B and C.
(iv) Mark the point P which is equidistant from AB, BC, and also equidistant from B and C. Measure and record the length of PB.
Ruler and compasses only may be used in this question. All constructions lines and arcs must be clearly shown, and the be sufficient length and clarity to permit assessment:
(i) Construct a triangle ABC, in which AB = 9 cm, BC = 10 cm and angle ABC = 45°.
(ii) Draw a circle, with center A and radius 2.5 cm. Let it meet AB at D.
(iii) Construct a circle to touch the circle with center A externally at D and also to touch the line BC.
