Advertisements
Advertisements
Question
Repeat Question 6, if `overline"AB"` happens to be a diameter.
Solution
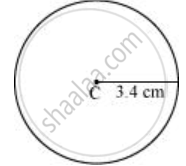
- Mark any point C on the sheet.
- By adjusting the compasses up to 3.4 cm and by putting the pointer of the compasses at point C, turn the compasses slowly to draw the circle. It is the required circle of 3.4 cm radius.
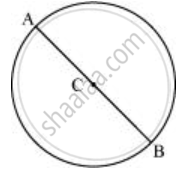
- Mark any diameter `overline"AB"` in the circle.
-
Now, taking A and B as centres, draw arcs on both sides of `overline"AB"` taking radius more than `overline"AB"`. Let these intersect each other at D and E.

- Join DE, which is the perpendicular bisector of AB.

It can be observed that `overline"DE"` is passing through the centre C of the circle.
APPEARS IN
RELATED QUESTIONS
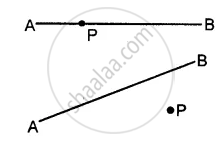
In each of the following, draw perpendicular through point P to the line segment AB :
(i)

(ii)

(iii)
Draw a line segment AB = 5.5 cm. Mark a point P, such that PA = 6 cm and PB = 4.8 cm. From point P, draw a perpendicular to AB.
Draw a line segment of given length and construct a perpendicular bisector to line segment using scale and compass
8 cm
Draw a line segment of length 7 cm. Draw its perpendicular bisector, using ruler and compasses.
Draw a line segment of length 6.5 cm and divide it into four equal parts, using ruler and compasses.
Copy figure on your notebook and draw a perpendicular from P to line m using (i) set squares (ii) protractor (iii) ruler and compass. How many such perpendicular are you able to draw?
Bisect a right angle, using ruler and compasses. Measure each part. Bisect each of these parts. What will be the measure of each of these parts?
Draw a line segment of length 10 cm. Divide it into four equal parts. Measure each of these parts.
Draw the perpendicular bisector of `overline"XY"` whose length is 10.3 cm.
- Take any point P on the bisector drawn. Examine whether PX = PY
-
If M is the midpoint of `overline"XY"`, what can you say about the lengths MX and XY?
Draw a line segment of length 12.8 cm. Using compasses, divide it into four equal parts. Verify by actual measurement.
