Advertisements
Advertisements
Question
Show that the following points are collinear:
A(-5,1), B(5, 5) and C(10, 7)
Solution
`"Let " A(x_1=-5,y_1=1), B(x_2=5,y_2=5) and C(x_3= 10,y_3=7)`be the given points.
` Now , x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2)`
`=(-5)(5-7)+5(7-1)+10(1-5)`
`=-5(-2)+5(6)+10(-4)`
`=10+30-40`
= 0
Hence, the given points are collinear.
APPEARS IN
RELATED QUESTIONS
Find the area of the quadrilateral whose vertices, taken in order, are (-4, -2), (-3, -5), (3, -2) and (2, 3).
Let A (4, 2), B (6, 5) and C (1, 4) be the vertices of ΔABC.
(i) The median from A meets BC at D. Find the coordinates of point D.
(ii) Find the coordinates of the point P on AD such that AP: PD = 2:1
(iii) Find the coordinates of point Q and R on medians BE and CF respectively such that BQ: QE = 2:1 and CR: RF = 2:1.
(iv) What do you observe?
(v) If A(x1, y1), B(x2, y2), and C(x3, y3) are the vertices of ΔABC, find the coordinates of the centroid of the triangle.
Find the area of a triangle with vertices at the point given in the following:
(−2, −3), (3, 2), (−1, −8)
Find values of k if area of triangle is 4 square units and vertices are (k, 0), (4, 0), (0, 2)
A(7, -3), B(5,3) and C(3,-1) are the vertices of a ΔABC and AD is its median. Prove that the median AD divides ΔABC into two triangles of equal areas.
Find the value of k so that the area of the triangle with vertices A (k+1, 1), B(4, -3) and C(7, -k) is 6 square units
The points (0, 5), (0, –9) and (3, 6) are collinear.
If the points A(1, 2), O(0, 0) and C(a, b) are collinear, then ______.
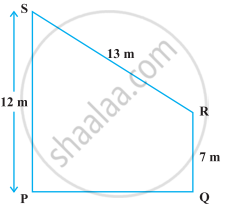
Find the area of the trapezium PQRS with height PQ given in the following figure.
If (a, b), (c, d) and (e, f) are the vertices of ΔABC and Δ denotes the area of ΔABC, then `|(a, c, e),(b, d, f),(1, 1, 1)|^2` is equal to ______.
