Advertisements
Advertisements
Question
Let A (4, 2), B (6, 5) and C (1, 4) be the vertices of ΔABC.
(i) The median from A meets BC at D. Find the coordinates of point D.
(ii) Find the coordinates of the point P on AD such that AP: PD = 2:1
(iii) Find the coordinates of point Q and R on medians BE and CF respectively such that BQ: QE = 2:1 and CR: RF = 2:1.
(iv) What do you observe?
(v) If A(x1, y1), B(x2, y2), and C(x3, y3) are the vertices of ΔABC, find the coordinates of the centroid of the triangle.
Solution
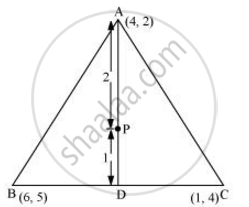
(i) Median AD of the triangle will divide the side BC into two equal parts.
Therefore, D is the mid-point of side BC
Coordinate of D = ` ((6+1)/2, (5+4)/2) = (7/2, 9/2)`
(ii) Point P divides the side AD in a ratio 2:1.
Coordinate of P =`((2xx7/2+1xx4)/(2+1), (2xx9/2+1xx2)/(2+1))= (11/3, 11/3)`
(iii) Median BE of the triangle will divide the side AC into two equal parts.
Therefore, E is the mid-point of the side AC.
Coordinate of E =` ((4+1)/2,(2+4)/2) = (5/2, 3)`
Point Q divides the side BE in a ratio 2:1.
Coordinate of Q =`((2xx5/2+1xx6)/(2+1), (2xx3+1xx5)/(2+1)) = (11/3,11/3)`
Median CF of the triangle will divide the side AB in two equal parts. Therefore, F is the mid-point of side AB
Coordinate of F =`((4+6)/2, (2+5)/2) = (5, 7/2)`
Point R divides the side CF in a ratio 2:1.
Coordinate of R =`((2xx5+1xx1)/(2+1),(2xx7/2+1xx4)/(2+1)) = (11/3, 11/3)`
(iv) It can be observed that the coordinates of point P, Q, R are the same.
Therefore, all these are representing the same point on the plane i.e., the centroid of the triangle.
(v) Consider a triangle, ΔABC, having its vertices as A(x1, y1), B(x2, y2), and C(x3, y3).
The median AD of the triangle will divide the side BC into two equal parts. Therefore, D is the mid-point of side BC.
Coordinate of D =`((x_2+x_3)/2,(y_2+y_3)/2)`
Let the centroid of this triangle be O.
Point O divides the side AD in a ratio of 2:1.
Coordinate of O = `((2xx(x_2+x_3)/2+1xxx_1)/(2+1), (2xx(y_2+y_3)/2+1xxy_1)/(2+1))`
`= ((x_1+x_2+x_3)/3, (y_1+y_2+y_3)/3)`
APPEARS IN
RELATED QUESTIONS
Find the values of k so that the area of the triangle with vertices (1, -1), (-4, 2k) and (-k, -5) is 24 sq. units.
Prove that (2, -2) (-2, 1) and (5, 2) are the vertices of a right-angled triangle. Find the area of the triangle and the length of the hypotenuse.
Prove that the points A(2, 4), b(2, 6) and (2 +`sqrt(3)` ,5) are the vertices of an equilateral triangle
Show that the following points are collinear:
(i) A(2,-2), B(-3, 8) and C(-1, 4)
Find the value of x for which points A(x, 2), B(-3, -4) and C(7, -5) are collinear.
Using determinants, find the values of k, if the area of triangle with vertices (–2, 0), (0, 4) and (0, k) is 4 square units.
Find the value of p for which the points (−5, 1), (1, p) and (4, −2) are collinear.
The table given below contains some measures of the right angled triangle. Find the unknown values.
| Base | Height | Area |
| 20 cm | 40 cm | ? |
The area of a triangle with vertices A(3, 0), B(7, 0) and C(8, 4) is ______.
The area of a trapezium is 475 cm2 and the height is 19 cm. Find the lengths of its two parallel sides if one side is 4 cm greater than the other.
