Advertisements
Advertisements
Question
Using determinants, find the values of k, if the area of triangle with vertices (–2, 0), (0, 4) and (0, k) is 4 square units.
Solution
A(ΔABC ) = 4
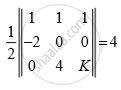
1(0-0)-1(-2K)+1(-8-0)=±8
2K - 8 = ±8
2K = ±8 + 8
∴ K =`16/2` or K=`0/2 rArr` K =8 or K = 0
APPEARS IN
RELATED QUESTIONS
A(4, - 6), B(3,- 2) and C(5, 2) are the vertices of a 8 ABC and AD is its median. Prove that the median AD divides Δ ABC into two triangles of equal areas.
Find the area of the triangle ABC with A(1, −4) and mid-points of sides through A being (2, −1) and (0, −1).
Find the area of the triangle PQR with Q(3,2) and the mid-points of the sides through Q being (2,−1) and (1,2).
Prove that the points (2, – 2), (–3, 8) and (–1, 4) are collinear
For what value of k are the points (k, 2 – 2k), (–k + 1, 2k) and (–4 – k, 6 – 2k) are collinear ?
Find the area of the triangle whose vertices are: (2, 3), (-1, 0), (2, -4)
Find the area of a triangle with vertices at the point given in the following:
(−2, −3), (3, 2), (−1, −8)
Show that points A (a, b + c), B (b, c + a), C (c, a + b) are collinear.
Find values of k if area of triangle is 4 square units and vertices are (−2, 0), (0, 4), (0, k)
If A(–5, 7), B(–4, –5), C(–1, –6) and D(4, 5) are the vertices of a quadrilateral, find the area of the quadrilateral ABCD
Find the area of the quadrilaterals, the coordinates of whose vertices are
(1, 2), (6, 2), (5, 3) and (3, 4)
Prove that the points (2a, 4a), (2a, 6a) and `(2a + sqrt3a, 5a)` are the vertices of an equilateral triangle.
Prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another
prove that the points A (7, 10), B(-2, 5) and C(3, -4) are the vertices of an isosceles right triangle.
Show that the following points are collinear:
A(5,1), B(1, -1) and C(11, 4)
Find the value of y for which the points A(-3, 9), B(2,y) and C(4,-5) are collinear.
If the points P(-3, 9), Q(a, b) and R(4, -5) are collinear and a+b=1, find the value of a and b.
Find the value(s) of k so that the quadratic equation x2 − 4kx + k = 0 has equal roots.
Find the value of p for which the points (−5, 1), (1, p) and (4, −2) are collinear.
Using integration, find the area of triangle ABC, whose vertices are A(2, 5), B(4, 7) and C(6, 2).
Find the area of the following triangle:

In ∆PQR, PR = 8 cm, QR = 4 cm and PL = 5 cm. 
Find:
(i) the area of the ∆PQR
(ii) QM.
If the co-ordinates of the vertices of an equilateral triangle with sides of length ‘a’ are (x1, y1), (x2, y2), (x3, y3), then `|(x_1, y_1, 1),(x_2, y_2, 1),(x_3, y_3, 1)|^2 = (3"a"^4)/4`
If the points (a1, b1), (a2, b2) and(a1 + a2, b1 + b2) are collinear, then ____________.
The area of a triangle with vertices (a, b + c), (b, c + a) and (c, a + b) is ______.
The points (0, 5), (0, –9) and (3, 6) are collinear.
If the points A(1, 2), O(0, 0) and C(a, b) are collinear, then ______.
If area of a triangular piece of cardboard is 90 cm2, then the length of altitude corresponding to 20 cm long base is ______ cm.
Find the missing value:
| Base | Height | Area of Triangle |
| ______ | 31.4 mm | 1256 mm2 |
