Advertisements
Advertisements
Question
Show that points A (a, b + c), B (b, c + a), C (c, a + b) are collinear.
Solution
It is known that the vertices of the triangles A (a, b + c), B (b, c + a) and C (c, a + b)
`Delta` area of `= Delta = 1/2 abs (("x"_1,"y"_1,1),("x"_2,"y"_2,1),("x"_3,"y"_3,1))`
x1 = a,y1 = b + c, x2 = b, y2 = c + a, x3 = c, y3 = a + b
`= 1/2 abs (("a", "b + c", 1),("b", "c + a",1),("c", "a + b", 1)) ...("C"_1 -> "C"_1 + "C"_2)`
`= 1/2 abs (("a + b + c", "b + c", 1),("a + b + c", "c + a", 1),("a + b + c", "a + b", 1))`
`= 1/2 ("a + b + c") abs ((1, "b + c", 1),(1, "c + a", 1),(1, "a + b", 1))`
`= 1/2 ("a + b + c") xx 0 ...("C"_1 "and" "C"_2 "are the same")`
`Delta` area of = 0
Hence, points A, B, C are collinear.
APPEARS IN
RELATED QUESTIONS
Find the relation between x and y if, the points A(x, y), B(-5, 7) and C(-4, 5) are collinear.
Find the values of k so that the area of the triangle with vertices (1, -1), (-4, 2k) and (-k, -5) is 24 sq. units.
Find the area of the triangle ABC with A(1, −4) and mid-points of sides through A being (2, −1) and (0, −1).
If A(−4, 8), B(−3, −4), C(0, −5) and D(5, 6) are the vertices of a quadrilateral ABCD, find its area.
If the points P(–3, 9), Q(a, b) and R(4, – 5) are collinear and a + b = 1, find the values of a and b.
Find the area of the quadrilateral ABCD whose vertices are respectively A(1, 1), B(7, –3), C(12, 2) and D(7, 21).
Find the area of the triangle whose vertices are: (2, 3), (-1, 0), (2, -4)
Find the area of the triangle whose vertices are: (–5, –1), (3, –5), (5, 2)
The two opposite vertices of a square are (− 1, 2) and (3, 2). Find the coordinates of the other two vertices.
Find the area of a triangle with vertices at the point given in the following:
(1, 0), (6, 0), (4, 3)
Find values of k if area of triangle is 4 square units and vertices are (k, 0), (4, 0), (0, 2)
The area of a triangle is 5 sq units. Two of its vertices are (2, 1) and (3, –2). If the third vertex is (`7/2`, y). Find the value of y
ΔABC is isosceles with AB = AC = 7.5 cm and BC = 9 cm (see the given figure). The height AD from A to BC, is 6 cm. Find the area of ΔABC. What will be the height from C to AB i.e., CE?

If A(–5, 7), B(–4, –5), C(–1, –6) and D(4, 5) are the vertices of a quadrilateral, find the area of the quadrilateral ABCD
The four vertices of a quadrilateral are (1, 2), (−5, 6), (7, −4) and (k, −2) taken in order. If the area of the quadrilateral is zero, find the value of k.
Prove that (2, -2) (-2, 1) and (5, 2) are the vertices of a right-angled triangle. Find the area of the triangle and the length of the hypotenuse.
If `a≠ b ≠ c`, prove that the points (a, a2), (b, b2), (c, c2) can never be collinear.
Prove that the points (a, b), (a1, b1) and (a −a1, b −b1) are collinear if ab1 = a1b.
If G be the centroid of a triangle ABC and P be any other point in the plane, prove that PA2+ PB2 + PC2 = GA2 + GB2 + GC2 + 3GP2.
prove that the points A (7, 10), B(-2, 5) and C(3, -4) are the vertices of an isosceles right triangle.
Find the third vertex of a ΔABC if two of its vertices are B(-3,1) and C (0,-2) and its centroid is at the origin
Find the area of ΔABC whose vertices are:
A(-5,7) , B (-4,-5) and C (4,5)
A(7, -3), B(5,3) and C(3,-1) are the vertices of a ΔABC and AD is its median. Prove that the median AD divides ΔABC into two triangles of equal areas.
A(6,1) , B(8,2) and C(9,4) are the vertices of a parallelogram ABCD. If E is the midpoint of DC, find the area of ΔADE
For what value of y, are the points P(1, 4), Q(3,y) and R(-3, 16) are collinear ?
Find a relation between x and y, if the points A(x, y), B(-5, 7) and C(-4, 5) are collinear.
Find the area of ΔABC with vertices A(0, -1), B(2,1) and C(0, 3). Also, find the area of the triangle formed by joining the midpoints of its sides. Show that the ratio of the areas of two triangles is 4:1.
If the area of triangle ABC formed by A(x, y), B(1, 2) and C(2, 1) is 6 square units, then prove that x + y = 15 ?
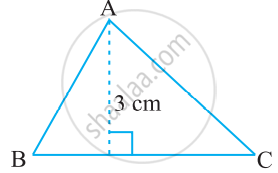
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
If Δ = `|(1, x, x^2),(1, y, y^2),(1, z, z^2)|`, Δ1 = `|(1, 1, 1),(yz, zx, xy),(x, y, z)|`, then prove that ∆ + ∆1 = 0.
If the co-ordinates of the vertices of an equilateral triangle with sides of length ‘a’ are (x1, y1), (x2, y2), (x3, y3), then `|(x_1, y_1, 1),(x_2, y_2, 1),(x_3, y_3, 1)|^2 = (3"a"^4)/4`
The area of ∆ABC is 8 cm2 in which AB = AC = 4 cm and ∠A = 90º.
The area of a trapezium is 475 cm2 and the height is 19 cm. Find the lengths of its two parallel sides if one side is 4 cm greater than the other.
If area of a triangular piece of cardboard is 90 cm2, then the length of altitude corresponding to 20 cm long base is ______ cm.
Observe all the four triangles FAB, EAB, DAB and CAB as shown in the given figure.

- All triangles have the same base and the same altitude.
- All triangles are congruent.
- All triangles are equal in area.
- All triangles may not have the same perimeter.
In the given figure, ratio of the area of triangle ABC to the area of triangle ACD is the same as the ratio of base BC of triangle ABC to the base CD of ΔACD.