Advertisements
Advertisements
Question
Find the area of the trapezium PQRS with height PQ given in the following figure.
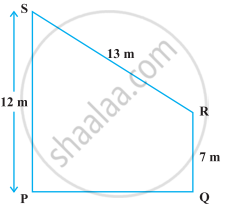
Solution
We have, trapezium PQRS, in which draw a line RT perpendicular to PS.
Where, side, ST = PS – TP = 12 – 7 = 5 m. ...[∵ TP = PQ = 7 m]
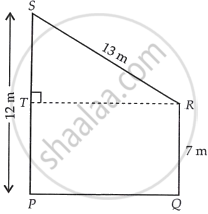
In right angled ΔSTR,
(SR)2 = (ST)2 + (TR)2 ...[By using Pythagoras theorem]
⇒ (13)2 = (5)2 + (TR)2
⇒ (TR)2 = 169 – 25
⇒ (TR)2 = 144
∴ TR = 12 m ...[Taking positive square root because length is always positive]
Now, area of ΔSTR = `1/2 xx TR xx TS` ...[∵ Area of triangle = `1/2` (base × height)]
= `1/2 xx 12 xx 5`
= 30 m2
Now, area of rectangle PQRT = PQ × RQ ...[∵ Area of a rectangle = length × breadth]
= 12 × 7
= 84 m2 ...[∵ PQ = TR = 12 m]
∴ Area of trapezium = Area of ΔSTR + Area of rectangle PQRT
= 30 + 84
= 114 m2
Hence, the area of trapezium is 114 m2.
APPEARS IN
RELATED QUESTIONS
The perimeter of a right triangle is 60 cm. Its hypotenuse is 25 cm. Find the area of the triangle.
Find the area of the triangle formed by joining the mid-points of the sides of the triangle whose vertices are (0, -1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle
ABCD is a rectangle formed by the points A(-1, -1), B(-1, 4), C(5, 4) and D(5, -1). P, Q, R and S are the midpoints of AB, BC, CD and DA respectively. Is the quadrilateral PQRS a square? a rectangle? or a rhombus? Justify your answer.
Find values of k if area of triangle is 4 square units and vertices are (−2, 0), (0, 4), (0, k)
Prove that (2, -2) (-2, 1) and (5, 2) are the vertices of a right-angled triangle. Find the area of the triangle and the length of the hypotenuse.
Show that the points are the vertices of an isosceles right triangle.
If the centroid of ΔABC having vertices A (a,b) , B (b,c) and C (c,a) is the origin, then find the value of (a+b+c).
Show that ∆ ABC with vertices A (–2, 0), B (0, 2) and C (2, 0) is similar to ∆ DEF with vertices D (–4, 0), F (4, 0) and E (0, 4) ?
Using integration, find the area of triangle ABC, whose vertices are A(2, 5), B(4, 7) and C(6, 2).
The area of the triangle ABC with the vertices A(-5, 7), B(-4, -5) and C(4, 5) is ______.
