Advertisements
Advertisements
Question
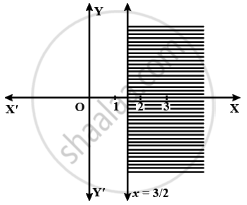
Solve graphically: 2x – 3 ≥ 0
Solution
Consider the line whose equation is 2x – 3 ≥ 0, i.e. x = `3/2`
This represents a line parallel to Y-axis passing through the point `(3/2, 0)`.
Draw the line x = `3/2`.
To find the solution set, we have to check the position of the origin (0, 0).
When x = 0, 2x – 3 = 2 × 0 – 3 = – 3 `cancel>=` 0
∴ The coordinates of the origin does not satisfy the given inequality.
∴ The solution set consists of the line x = `3/2` and the non-origin side of the line which is shaded in the graph.
RELATED QUESTIONS
Solve graphically: x ≥ 0
Solve graphically : y ≥ 0
Solve graphically : x ≤ 0
Solve graphically : y ≤ 0
Solve graphically: x ≤ 0 and y ≥ 0
Solve graphically : x ≤ 0 and y ≤ 0
Solve graphically : 2y – 5 ≥ 0
Solve graphically : 3x + 4 ≤ 0
Solve graphically : 5y + 3 ≤ 0
Solve graphically : x +2y ≤ 6
Solve graphically: 3x + 2y ≥ 0
Solve graphically : 5x – 3y ≤ 0
Solve graphically : 2x + y ≥ 2 and x – y ≤ 1
Solve graphically : x + y ≥ 6 and x + 2y ≤ 10
Solve graphically : 2x + 3y≤ 6 and x + 4y ≥ 4
Solve graphically : 2x + y ≥ 5 and x – y ≤ 1
The corner points of the feasible solutions are (0, 0) (3, 0) (2, 1) (0, 7/3) the maximum value of Z = 4x + 5y is
The half plane represented by 4x + 3y >14 contains the point
A solution set of the inequality x ≥ 0
Check the ordered points (1, −1), (2, −1) is a solution of 2x + 3y − 6 ≤ 0
Show the solution set of inequations 4x – 5y ≤ 20 graphically
For the constraint of a linear optimizing function z = 3x1 + 11x2, given by 2x1 + x2 ≤ 2, 4x1 + x2 ≥ 4 and x1, x2 ≥ 0
Let p and q be the statements:
p: 3x3 + 8y3 ≥ 15, q: 5x + 2y < 11
Then, which of the following is true?
Solution of the LPP minimize z = 7x + 2y subject to x + y ≥ 60, x - 2y ≥ 0, x + 2y ≤ 120, x, y ≥ 0 is ______
The maximum value of z = 7x + 6y.
Subject to the constraints x ≤ 45, y ≤ 55 and x ≥ 0, y ≥ 0 is ______.
Region represented by the inequalities x ≥ 0, y ≤ 0 is ______.
If S1 and S2 are respectively the sets of local minimum and local maximum points of the function, f(x) = 9x4 + 12x3 - 36x2 + 25, x ∈ R, then ______
Solution set of the inequality y ≥ 0 is ______.
The set of real x satisfying the inequality `(5 - 2x)/3 ≤ x/6 - 5` is [a, ∞). The value of ‘a’ is ______.
Which of the following linear inequalities satisfy the shaded region of the given figure?

The object function z = 4x1 + 5x2, subject to 2x1 + x2 ≥ 7, 2x1 + 3x2 ≤ 15, x2 ≤ 3, x1, x2 ≥ 0 has minimum value at the point is ______.
The objective function of LPP defined over the convex set attains it optimum value at ______.
