Advertisements
Advertisements
Question
The diameter of a wheel is 1.4m. How many revolutions does it make in moving a distance of 2.2 kms?
Solution
Diameter of the wheel = 1. 4 m
Circumference = 2 π r = π d
`= 22/7 xx 1.4`
= 4.4 m
Circumference= 8.8 m
Distance travelled = 2.2 km = 2200 m
No. of revolutions = `2200/4.4 = 500`
Wheel makes 500 revolutions in travelling 2.2 km
APPEARS IN
RELATED QUESTIONS
The length of minute hand of a clock is 14 cm. Find the area swept by the minute hand in one minute. (Use π = 22/7)
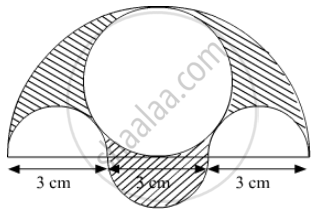
Three semicircles each of diameter 3 cm, a circle of diameter 4.5 cm and a semicircle of radius 4.5 cm are drawn in the given figure. Find the area of the shaded region
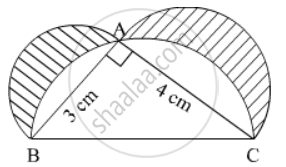
In the given figure, ∆ ABC is a right-angled triangle in which ∠ A is 90°. Semicircles are drawn on AB, AC and BC as diameters. Find the area of the shaded region
The sum of the radii of two circles is 140 cm and the difference of their circumferences in 88 cm. Find the diameters of the circles.
Find in terms of x the length of the arc that subtends an angle of 30°, at the centre of circle of radius 4 cm.
Find the angle subtended at the centre of circle of radius ‘a’ cm by an arc of length
`(api)/4` 𝑐𝑚
Choose the correct alternative answer for the following question.
Four equal circles, each of radius a units, touch each other. Show that the area between them is `(6/7"a"^2)` sq units.
The perimeter of a sector of a circle of radius 5.6 cm is 27.2 cm. Find the area of the sector.
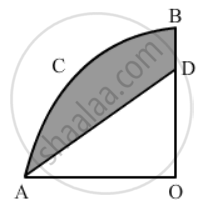
OACB is a quadrant of a circle with centre O and its radius is 3.5 cm. If OD = 2 cm. find the area of
- quadrant OACB
- the shaded region.
A wire is in the form of a circle of radius 42 cm. It is bent into a square.
Determine the side of the square and compare the area of the regions enclosed in two cases.
The speed of the car is 66 km/hour. If each wheel of the car is 140 cm in diameter, find the number of revolutions made by each wheel per minute.
The diameter of the wheel of a cart is 1.05 m. How much distance will the cart cover in 1000 rotations of the wheel?
Find the area and perimeter of the following semicircles: Diameter = 5.6cm
The circumference of a circle exceeds its diameter by 450 cm. Find the area of the circle.
Find the radius and area of the circle which has circumference equal to the sum of circumferences of the two circles of radii 3 cm and 4 cm respectively.
A cart wheel makes 9 revolutions per second. If the diameter of the wheel is 42cm, find its speed in km per hour. (Answer correct to the nearest km)
A race track is in the form of a circular ring whose outer and inner circumferences are 396 m and 352 m respectively. The width of the track is ____________.
The numerical value of the area of a circle is greater than the numerical value of its circumference. Is this statement true? Why?
