Advertisements
Advertisements
Question
The perimeter of a semicircular plate is 108 cm. find its area.
Solution
The perimeter of a semi-circular plate = 108 cm
⇒ πr + 2r = 108 ...(r is radius of a circle )
⇒ r ( π + 2 ) = 108
⇒ r = ` (108)/( π + 2) = (108)/(22/7 + 2) = (108 xx 7) / (36) = 21 "cm"`
Now, area of a semi-ccircular pleate = `[πr^2]/2 = [22/7 xx 21 xx 21]/2 = 693 "cm"^2`
APPEARS IN
RELATED QUESTIONS
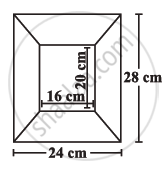
Diagram of the adjacent picture frame has outer dimensions = 24 cm × 28 cm and inner dimensions 16 cm × 20 cm. Find the area of each section of the frame, if the width of each section is same.
Calculate the area of quadrilateral ABCD in which AB = 32 cm, AD = 24 cm ∠A = 90° and BC = CD = 52 cm.
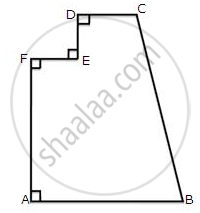
The figure given below shows the cross-section of a concrete structure. Calculate the area of cross-section if AB = 1.8 cm, CD = 0.6 m, DE = 0.8 m, EF = 0.3 m and AF = 1.2 m.
The length of a rectangular verandah is 3 m more than its breadth. The numerical value of its area is equal to the numerical value of its perimeter.
(i) Taking x as the breadth of the verandah, write an equation in x that represents the above statement
(ii) Solve the equation obtained in (i) above and hence find the dimensions of the verandah.
Find the area and perimeter of a square plot of land, the length of whose diagonal is 15 meters. Given your answer correct to 2 places of decimals.
A triangle and a parallelogram have the same base and the same area. If the side of the triangle is 26 cm, 28 cm, and 30 cm and the parallelogram stands on the base 28 cm, find the height of the parallelogram.
Using the information in the following figure, find its area.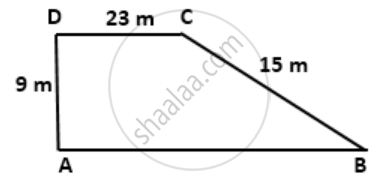
Find the diagonal of a quadrilateral whose area is 756cm2 and the perpendicular from the opposite vertices are 17cm and 19cm.
Let P(11, 7), Q(13.5, 4) and R(9.5, 4) be the midpoints of the sides AB, BC and AC respectively of ∆ABC. Find the coordinates of the vertices A, B and C. Hence find the area of ∆ABC and compare this with area of ∆PQR.
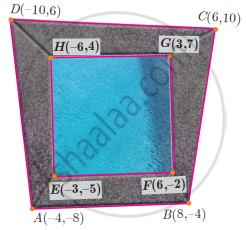
The quadrilateral swimming pool shown is surrounded by concrete patio. Find the area of the patio