Advertisements
Advertisements
Question
The ratio between the exterior angle and the interior angle of a regular polygon is 1 : 4. Find the number of sides in the polygon.
Solution
Let exterior angle = x° & interior angle = 4x°
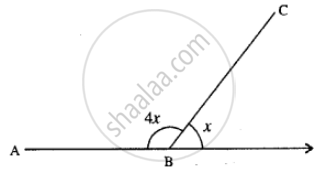
∴ 4x + x = 180°
5x = 180°
x = 36°
∴ Each exterior angle = 36°
Let no.of sides = n
∴ `360^circ/"n" = 36^circ`
n = `360^circ/36^circ`
n = 10
APPEARS IN
RELATED QUESTIONS
Fill in the blanks :
In case of regular polygon, with :
| No.of.sides | Each exterior angle | Each interior angle |
| (i) ___8___ | _______ | ______ |
| (ii) ___12____ | _______ | ______ |
| (iii) _________ | _____72°_____ | ______ |
| (iv) _________ | _____45°_____ | ______ |
| (v) _________ | __________ | _____150°_____ |
| (vi) ________ | __________ | ______140°____ |
Find the number of sides in a regular polygon, if its interior angle is: 135°
Find the number of sides in a regular polygon, if its exterior angle is : `1/3` of right angle
Is it possible to have a regular polygon whose each exterior angle is: 80°
The measure of each interior angle of a regular polygon is five times the measure of its exterior angle. Find :
(i) measure of each interior angle ;
(ii) measure of each exterior angle and
(iii) number of sides in the polygon.
The ratio between the interior angle and the exterior angle of a regular polygon is 2: 1. Find:
(i) each exterior angle of the polygon ;
(ii) number of sides in the polygon.
The sum of interior angles of a regular polygon is twice the sum of its exterior angles. Find the number of sides of the polygon.
The difference between the exterior angles of two regular polygons, having the sides equal to (n – 1) and (n + 1) is 9°. Find the value of n.
If the difference between the exterior angle of a 'n' sided regular polygon and an (n + 1) sided regular polygon is 12°, find the value of n.
Three of the exterior angles of a hexagon are 40°, 51 ° and 86°. If each of the remaining exterior angles is x°, find the value of x.
