Advertisements
Advertisements
Question
Two discs of moments of inertia I1 and I2 about their respective axes (normal to the disc and passing through the centre), and rotating with angular speeds ω1 and ω2 are brought into contact face to face with their axes of rotation coincident. (a) What is the angular speed of the two-disc system? (b) Show that the kinetic energy of the combined system is less than the sum of the initial kinetic energies of the two discs. How do you account for this loss in energy? Take ω1 ≠ ω2.
Solution
a)Moment of inertia of disc `I = I_1`
Angular speed of disc `I = omega_1`
Angular speed of disc II = `I_2`
Angular momentum of disc II = `omega_1`
Angular momentum of disc I = `L_1 = I_1omega_1`
Angular momentum of disc II, `L_2 = I_2omega2`
Total initial angular momentum, `L_1 = I_1omega_1 + I_2omega_2`
When the two discs are joined together, their moments of inertia get added up.
Moment of inertia of the system of two discs, I = `I_1 + I_2`
Let ω be the angular speed of the system
Total final angular momentum, `L_f = (I_1 + I_2) omega`
Using the law of conservation of angular momentum, we have:
`L_i = L_f`
`I_1omega_1 + I_2omega_2 = (I_1+_ I_2)omega`
`:. omega = (I_1omega_1 + I_2omega_2)/(I_1+I_2)`
b) Kinetic energy of disc I, `E_1 = 1/2 I_1omega_1^2`
Kinetic energy of disc II, `E_2 = 1/2 I_2omega_2^2`
Total initial kinetic energy, `E_i = 1/2 (I_1omegha_1^2 + I_2omega_2^2)`
When the discs are joined, their moments of inertia get added up.
Moment of inertia of the system, `I=I_1+I_2`
Angular speed of the system = ω
Final kinetic energy Ef:
`=1/2(I_1 +I_2)omega^2`
`=1/2 (I_1+I_2)((I_1omega_1 + I_2omega_2)/(I_1+I_2))^2 = 1/2 (I_1omega_1 + I_2omega_2)/(I_1+I_2)`
`:.E_i - E_f`
`=1/2 (I_1omega_1^2 + I_2omega_2^2) - (I_1omega_1 + I_1omega_2)^2/(2(I_1+I_2))`
`=1/2 I_1omega_1^2 + 1/2 I_2omega_2^2 - 1/2 (I_1^2omega_1^2)/(I_1+I_2) - 1/2 (I_2^2omega_2^2)/(2(I_1+I_2)) - 1/2 (2I_1I_2omega_1omega_2)/(2(I_1+I_2))`
`=1/(I_1+I_2) [1/2 I_1^2omega_1^2 + 1/2 I_1I_2omega_1^2 + 1/2 I_1I_2omega_2^2 + 1/2 I_2^2omega^2 - 1/2I_1^2 omega_1^2 - 1/2 I_2^2omega_2^2 - I_1I_2omega_1omega_2]`
`= (I_1I_2)/(2(I_1+I_2))[omega_1^2 + omega_2^2 - 2omega_1omega_2]`
= `(I_1I_2(omega_1-omega_2)^2)/(2(I_1+I_2))`
All the quantities on RHS are positive
`:.E_i - E_f > 0`
`E_i > E_f`
The loss of KE can be attributed to the frictional force that comes into play when the two discs come in contact with each other.
APPEARS IN
RELATED QUESTIONS
Given the moment of inertia of a disc of mass M and radius R about any of its diameters to be MR2/4, find its moment of inertia about an axis normal to the disc and passing through a point on its edge
Show that the child’s new kinetic energy of rotation is more than the initial kinetic energy of rotation. How do you account for this increase in kinetic energy?
A cylinder of mass 10 kg and radius 15 cm is rolling perfectly on a plane of inclination 30°. The coefficient of static friction µs = 0.25.
(a) How much is the force of friction acting on the cylinder?
(b) What is the work done against friction during rolling?
(c) If the inclination θ of the plane is increased, at what value of θ does the cylinder begin to skid, and not roll perfectly?
A body having its centre of mass at the origin has three of its particles at (a,0,0), (0,a,0), (0,0,a). The moments of inertia of the body about the X and Y axes are 0⋅20 kg-m2 each. The moment of inertia about the Z-axis
Solve the previous problem if the friction coefficient between the 2⋅0 kg block and the plane below it is 0⋅5 and the plane below the 4⋅0 kg block is frictionless.
A diver having a moment of inertia of 6⋅0 kg-m2 about an axis thorough its centre of mass rotates at an angular speed of 2 rad/s about this axis. If he folds his hands and feet to decrease the moment of inertia to 5⋅0 kg-m2, what will be the new angular speed?
A boy is seated in a revolving chair revolving at an angular speed of 120 revolutions per minute. Two heavy balls form part of the revolving system and the boy can pull the balls closer to himself or may push them apart. If by pulling the balls closer, the boy decreases the moment of inertia of the system from 6 kg-m2 to 2 kg-m2, what will be the new angular speed?
Moment of inertia (M.I.) of four bodies, having same mass and radius, are reported as :
I1 = M.I. of thin circular ring about its diameter,
I2 = M.I. of circular disc about an axis perpendicular to disc and going through the centre,
I3 = M.I. of solid cylinder about its axis and
I4 = M.I. of solid sphere about its diameter.
Then -
Consider a badminton racket with length scales as shown in the figure.
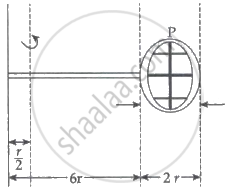
If the mass of the linear and circular portions of the badminton racket is the same (M) and the mass of the threads is negligible, the moment of inertia of the racket about an axis perpendicular to the handle and in the plane of the ring at, `r/2` distance from the ends A of the handle will be ______ Mr2.
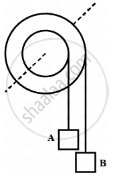
The figure shows a small wheel fixed coaxially on a bigger one of double the radius. The system rotates about the common axis. The strings supporting A and B do not slip on the wheels. If x and y be the distances travelled by A and B in the same time interval, then ______.