Advertisements
Advertisements
Question
Two lines l and m are perpendicular to the same line n. Are l and m perpendicular to each other? Give reason for your answer.
Solution
No.
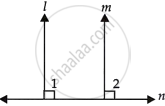
Given that the lines l and m are perpendicular to the line n.
∴ ∠1 = ∠2 = 90°
This shows that the corresponding angles are equal.
Thus, l || m.
APPEARS IN
RELATED QUESTIONS
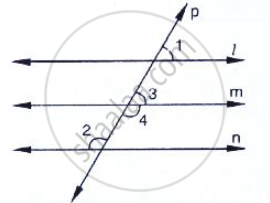
In the below fig, l, m and n are parallel lines intersected by transversal p at X, Y and Z
respectively. Find ∠1, ∠2 and ∠3.

In the below fig, if l || m || n and `∠`1 = 60°, find `∠`2.
Prove that the straight lines perpendicular to the same straight line are parallel to one
another.
Which of the following statement are true and false ? Give reason.
If two lines are intersected by a transversal, then corresponding angles are equal.
Which of the following statement are true and false ? Give reason.
If two parallel lines are intersected by a transversal, then the interior angles on the same side of the transversal are equal.
In the given figure, PQ || RS, ∠AEF = 95°, ∠BHS = 110° and ∠ABC = x°. Then the value of x is

In the given figure, if AB || CD, then x =

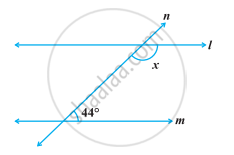
In the given figure, if lines l and m are parallel lines, then x =

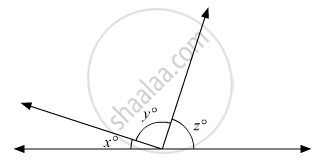
In the given figure, if \[\frac{y}{x} = 5\] and \[\frac{z}{x} = 4\] then the value of x isc
In the following figure, find the value of x for which the lines l and m are parallel.