Advertisements
Advertisements
Question
Two straight roads AB and CD cross each other at Pat an angle of 75° . X is a stone on the road AB, 800m from P towards B. BY taking an appropriate scale draw a figure to locate the position of a pole, which is equidistant from P and X, and is also equidistant from the roads.
Solution
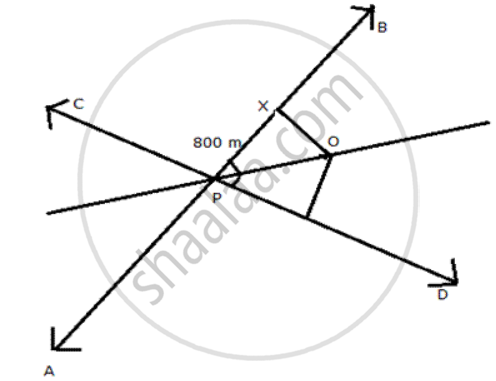
Steps of construction:
(i) Draw two lines AB and CD crossing at an angle of 75 °
(ii) Draw an angle bisector for ∠ BPD
(iii) Draw perpendicular from X on angle bisector meeting at 0.
(iv) From point Y, PX = PY, draw a perpendicular on angle bisector meeting at 0.
(v) 0 is the point which is equidistant from P, X and both the roads.
cos θ = `"hypotenuse"/"base"`
cos `75/2 = "PO"/"PX"`
cos (37.5) = `"PO"/800`
0.980243 = `"PO"/800`
PO = 784.19 m
APPEARS IN
RELATED QUESTIONS
Describe the locus of a point P, so that:
AB2 = AP2 + BP2,
where A and B are two fixed points.
Draw two intersecting lines to include an angle of 30°. Use ruler and compasses to locate points which are equidistant from these Iines and also 2 cm away from their point of intersection. How many such points exist?
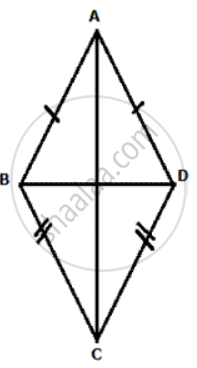
In given figure, ABCD is a kite. AB = AD and BC =CD. Prove that the diagona AC is the perpendirular bisector of the diagonal BD.
In given figure 1 ABCD is an arrowhead. AB = AD and BC = CD. Prove th at AC produced bisects BD at right angles at the point M

Draw and describe the lorus in the following cases:
The locus of points at a distance of 4 cm from a fixed line.
Describe completely the locus of point in the following cases:
Centre of a ball, rolling along a straight line on a level floor.
Describe completely the locus of points in the following cases:
Point in a plane equidistant from a given line.
Without using set squares or protractor construct:
(i) Triangle ABC, in which AB = 5.5 cm, BC = 3.2 cm and CA = 4.8 cm.
(ii) Draw the locus of a point which moves so that it is always 2.5 cm from B.
(iii) Draw the locus of a point which moves so that it is equidistant from the sides BC and CA.
(iv) Mark the point of intersection of the loci with the letter P and measure PC.
Use ruler and compasses only for the following questions:
Construct triangle BCP, when CB = 5 cm, BP = 4 cm, ∠PBC = 45°.
Complete the rectangle ABCD such that :
(i) P is equidistant from AB and BC and
(ii) P is equidistant from C and D. Measure and write down the length of AB.
Use ruler and compass to answer this question. Construct ∠ABC = 90°, where AB = 6 cm, BC = 8 cm.
- Construct the locus of points equidistant from B and C.
- Construct the locus of points equidistant from A and B.
- Mark the point which satisfies both the conditions (a) and (b) as 0. Construct the locus of points keeping a fixed distance OA from the fixed point 0.
- Construct the locus of points which are equidistant from BA and BC.
