Advertisements
Advertisements
Question
Using ruler and compasses only, construct a triangle ABC in which AB=S cm, BC=6 cm and CA=4.5 cm. Construct a circle passing through A, Band c.
Solution
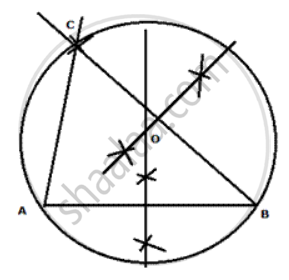
Steps of Construction:
(i) Draw line AB = S cm
(ii) At B, draw an arc with radius 6 cm
(iii) On the arc cut AC = 4.5 cm.
(iv) Join AC and BC.
(v) Draw perpendicular bisectors of AB and BC, which meet at O.
(vi) With O as centre and radius equal to the distance between O and the vertex of the triangle, draw a circle to pass through alI the three vertices of the triangle. (vii) The circle drawn is the required circle.
APPEARS IN
RELATED QUESTIONS
Using ruler and compasses only,
- Construct triangle ABC, having given BC = 7 cm, AB – AC = 1 cm and ∠ABC = 45°.
- Inscribe a circle in the ΔABC constructed in (i) above. Measure its radius.
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
- What do you call the point O?
- What is the relation between the distances OA, OB and OC?
- Does the perpendicular bisector of BC pass through O?
Contruct a ΔABC with BC = 6.5 cm, AB = 5.5 cm, AC = 5 cm. Construct the incircle of the triangle. Measure and record the radius of the incricle.
Draw a circle of radius 4.5 cm. Take a point Pon its circumference. Construct a tangent to the circle at P without using the centre.
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
What is the relation between the distances OA, OB and OC?
Construct a triangle ABC in which AB = 5 cm, BC = 6.8 cm and median AD = 4.4 cm. Draw incircle of this triangle.
In triangle ABC, ∠ABC = 90°, AB = 6 cm, BC = 7.2 cm and BD is perpendicular to side AC. Draw circumcircle of triangle BDC and then state the length of the radius of this circumcircle drawn.
Construct a Δ ABC with BC = 6.5 cm, AB = 5.5 cm, AC = 5 cm. Construct the incircle of the triangle. Measure and record the radius of the incircle.
Use ruler and compasses only for this question:
(i) Construct A ABC, where AB = 3.5 cm, BC = 6 cm and ∠ ABC = 60°.
(ii) Construct the locus of points inside the triangle which are equidistant from BA and BC.
(iii) Construct the locus of points inside the triangle which are equidistant from B and C.
(iv) Mark the point P which is equidistant from AB, BC, and also equidistant from B and C. Measure and record the length of PB.
Construct a ΔABC with base BC = 3.5 cm, vertical angle ∠ BAC = 45°, and median through the vertex A is 3.5 cm. Write also the steps of construction.
