Advertisements
Advertisements
Question
Write short notes on two springs connected in series.
Solution
- When two or more springs are connected in series, we can replace (by removing) all the springs in series with an equivalent spring (effective spring) whose net effect is the same as if all the springs are in series connection.
- Given the value of individual spring constants k1, k2, k3, ... (known quantity), we can establish a mathematical relationship to find out an effective (or equivalent) spring constant ks (unknown quantity).
- For simplicity, let us consider only two springs whose spring constants are k1 and k2 and which can be attached to a mass m as shown in Figure.
- The results thus obtained can be generalised for any number of springs in series.
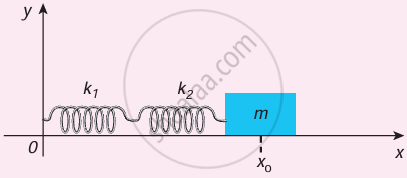
Springs are connected in series - Let F be the applied force towards right as shown in Figure. Since the spring constants for different springs are different and the connection points between them are not rigidly fixed, the strings can stretch in different lengths.
- Let x1 and x2 be the elongation of springs from their equilibrium position (un-stretched position) due to the applied force F. Then, the net displacement of the mass point is x = x1 + x2 ...(i)
- From Hooke’s law, the net force
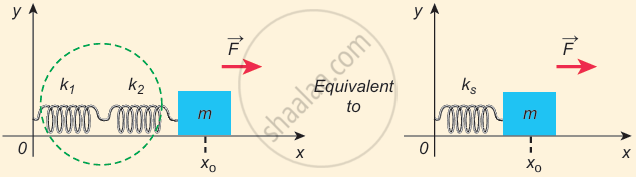
Effective spring constant in series connection
`F = -k_s(x_1 + x_2) => x_1 + x_2 = -F/K_s` ...(ii) - For springs in series connection
−k1 x1 = −k2 x2 = F
`=> x_1 = -F/k_1` and `x_2 = -F/k_2` ...(iii)
Therefore, substituting equation (iii) in equation (ii), the effective spring constant can be calculated as
`-F/k_1 - F/k_2 = -F/k_s`
`1/k_s = 1/k_1 + 1/k_2`
Or,
`k_s = (k_1k_2)/(k_1 + k_2)Nm^-1`
APPEARS IN
RELATED QUESTIONS
The time period of a particle in simple harmonic motion is equal to the smallest time between the particle acquiring a particular velocity \[\vec{v}\] . The value of v is
A pendulum clock that keeps correct time on the earth is taken to the moon. It will run
Select the correct statements.
(a) A simple harmonic motion is necessarily periodic.
(b) A simple harmonic motion is necessarily oscillatory.
(c) An oscillatory motion is necessarily periodic.
(d) A periodic motion is necessarily oscillatory.
Suppose a tunnel is dug along a diameter of the earth. A particle is dropped from a point, a distance h directly above the tunnel. The motion of the particle as seen from the earth is
(a) simple harmonic
(b) parabolic
(c) on a straight line
(d) periodic
A particle moves on the X-axis according to the equation x = x0 sin2 ωt. The motion is simple harmonic
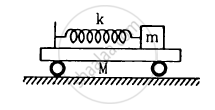
All the surfaces shown in figure are frictionless. The mass of the care is M, that of the block is m and the spring has spring constant k. Initially the car and the block are at rest and the spring is stretched through a length x0 when the system is released. (a) Find the amplitudes of the simple harmonic motion of the block and of the care as seen from the road. (b) Find the time period(s) of the two simple harmonic motions.
A small block oscillates back and forth on a smooth concave surface of radius R in Figure. Find the time period of small oscillation.

A simple pendulum of length l is suspended through the ceiling of an elevator. Find the time period of small oscillations if the elevator (a) is going up with and acceleration a0(b) is going down with an acceleration a0 and (c) is moving with a uniform velocity.
Define the time period of simple harmonic motion.
The displacement of a particle is represented by the equation `y = 3 cos (pi/4 - 2ωt)`. The motion of the particle is ______.
