Advertisements
Advertisements
Question
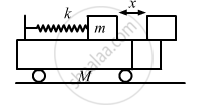
All the surfaces shown in figure are frictionless. The mass of the care is M, that of the block is m and the spring has spring constant k. Initially the car and the block are at rest and the spring is stretched through a length x0 when the system is released. (a) Find the amplitudes of the simple harmonic motion of the block and of the care as seen from the road. (b) Find the time period(s) of the two simple harmonic motions.
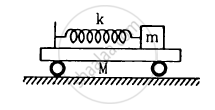
Solution
Let x1 and x2 be the amplitudes of oscillation of masses m and M respectively.
(a) As the centre of mass should not change during the motion, we can write:
mx1 = Mx2 \[\ldots(1)\]
Let k be the spring constant. By conservation of energy, we have:
\[\frac{1}{2}k x_0^2 = \frac{1}{2}k \left( x_1 + x_2 \right)^2 \ldots(2)\]
where x0 is the length to which spring is stretched.
From equation (2) we have,
\[x_0 = x_1 + x_2 \]
On substituting the value of x2 from equation (1) in equation (2), we get:
\[x_0 = x_1 + \frac{m x_1}{M}\]
\[ \Rightarrow x_0 = \left( 1 + \frac{m}{M} \right) x_1 \]
\[ \Rightarrow x_1 = \left( \frac{M}{M + m} \right) x_0\]
\[\text { Now }, x_2 = x_0 - x_1 \]
On substituting the value of x1 from above equation, we get:
\[\Rightarrow x_2 = x_0 \left[ 1 - \frac{M}{M + m} \right]\]
\[ \Rightarrow x_2 = \frac{m x_0}{M + m}\]
Thus, the amplitude of the simple harmonic motion of a car, as seen from the road is
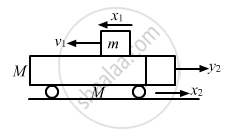
Let v1 and v2 be the velocities.
Using law of conservation of energy we have,
Now, from the principle of conservation of momentum, we have:
Mx2 = mx1
\[\Rightarrow x_1 = \left( \frac{M}{m} \right) x_2 . . . . \left( 4 \right)\]
\[M v_2 = m\left( v_1 - v_2 \right)\]
\[ \Rightarrow \left( v_1 - v_2 \right) = \left( \frac{M}{m} \right) v_2 . . . . \left( 5 \right)\]
Putting the above values in equation (3), we get:
\[\frac{1}{2}M v_2^2 + \frac{1}{2}m\frac{M^2}{m^2} v_2^2 + \frac{1}{2}k x_2^2 \left( 1 + \frac{M}{m} \right)^2 = \text { constant }\]
\[ \therefore M\left( 1 + \frac{M}{m} \right) v_2^2 + k\left( 1 + \frac{M}{m} \right) x_2^2 = \text { constant } \]
\[ \Rightarrow M v_2^2 + k\left( 1 + \frac{M}{m} \right) x_2^2 = \text { constant } \]
Taking derivative of both the sides, we get:
\[M \times 2 v_2 \frac{d v_2}{dt} + k\left( \frac{M + m}{m} \right)2 x_2 \frac{d x_2}{dt} = 0\]
\[ \Rightarrow m a_2 + k\left( \frac{M + m}{m} \right) x_2 = 0 \left[ \text { because }, v_2 = \frac{d x_2}{dt} \right]\]
\[\frac{a_2}{x_2} = \frac{- k\left( M + m \right)}{Mm} = \omega^2 \]
\[ \therefore \omega = \sqrt{\frac{k\left( M + m \right)}{Mm}}\]
\[\text { Therefore, time period }, T = 2\pi\sqrt{\frac{Mm}{k\left( M + m \right)}}\]
APPEARS IN
RELATED QUESTIONS
Which of the following relationships between the acceleration a and the displacement x of a particle involve simple harmonic motion?
(a) a = 0.7x
(b) a = –200x2
(c) a = –10x
(d) a = 100x3
Assuming the expression for displacement of a particle starting from extreme position, explain graphically the variation of velocity and acceleration w.r.t. time.
A body of mass 1 kg is made to oscillate on a spring of force constant 16 N/m. Calculate:
a) Angular frequency
b) frequency of vibration.
A block of known mass is suspended from a fixed support through a light spring. Can you find the time period of vertical oscillation only by measuring the extension of the spring when the block is in equilibrium?
The distance moved by a particle in simple harmonic motion in one time period is
A particle moves on the X-axis according to the equation x = A + B sin ωt. The motion is simple harmonic with amplitude
A pendulum clock keeping correct time is taken to high altitudes,
A particle moves in a circular path with a continuously increasing speed. Its motion is
Suppose a tunnel is dug along a diameter of the earth. A particle is dropped from a point, a distance h directly above the tunnel. The motion of the particle as seen from the earth is
(a) simple harmonic
(b) parabolic
(c) on a straight line
(d) periodic
A simple pendulum fixed in a car has a time period of 4 seconds when the car is moving uniformly on a horizontal road. When the accelerator is pressed, the time period changes to 3.99 seconds. Making an approximate analysis, find the acceleration of the car.
A uniform disc of mass m and radius r is suspended through a wire attached to its centre. If the time period of the torsional oscillations be T, what is the torsional constant of the wire?
A particle is subjected to two simple harmonic motions given by x1 = 2.0 sin (100π t) and x2 = 2.0 sin (120 π t + π/3), where x is in centimeter and t in second. Find the displacement of the particle at (a) t = 0.0125, (b) t = 0.025.
The length of a second’s pendulum on the surface of the Earth is 0.9 m. The length of the same pendulum on the surface of planet X such that the acceleration of the planet X is n times greater than the Earth is
Write short notes on two springs connected in series.
Write short notes on two springs connected in parallel.
What is meant by simple harmonic oscillation? Give examples and explain why every simple harmonic motion is a periodic motion whereas the converse need not be true.
Describe Simple Harmonic Motion as a projection of uniform circular motion.
A spring is stretched by 5 cm by a force of 10 N. The time period of the oscillations when a mass of 2 kg is suspended by it is ______
The displacement of a particle varies with time according to the relation y = a sin ωt + b cos ωt.
The velocities of a particle in SHM at positions x1 and x2 are v1 and v2 respectively, its time period will be ______.
