Advertisements
Advertisements
प्रश्न
A bullet of mass 10 g moving horizontally at a speed of 50√7 m/s strikes a block of mass 490 g kept on a frictionless track as shown in figure. The bullet remains inside the block and the system proceeds towards the semicircular track of radius 0.2 m. Where will the block strike the horizontal part after leaving the semicircular track?
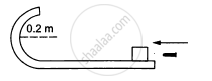
उत्तर
Given:
Mass of block = 490 gm
Initial speed of the block = 0
Mass of bullet = 10 gm
Initial speed of the bullet, v1 = \[50\sqrt{7} m/s\]
As the bullet gets embedded inside the block, this is an example of a perfectly inelastic collision.
Let the final velocity of the system (block and bullet) be VA.
Using the law of conservation of linear momentum, we get:
m1 + m2 × 0 = ( m1 + m2 ) VA
⇒10 × 10 - 3 × 507 + 490 × 10 - 3 × 0 = (490 + 10) × 10 - 3 × vA
∴ vA= \[\sqrt{7}\]
When the block loses contact at D, the component mg acts on it. Let the velocity at D be vB.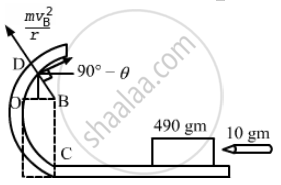
\[\frac{m( v_B )^2}{r} = mg\sin\theta\]
\[( v_B )^2 = gr \sin\theta . . . (1)\]
Using work energy theorem
\[\left( \frac{1}{2} \right)m v_B^2 - \left( \frac{1}{2} \right)m v_A^2 = - mg(0 . 2 + 0 . 2\sin\theta)\]
\[\left( \frac{1}{2} \right)gr\sin\theta - \left( \frac{1}{2} \right) \left( \sqrt{7} \right)^2 = g(0 . 2 + 0 . 2\sin\theta)\]
\[3 . 5 - \frac{1}{2} + 9 . 8 + 0 . 2 + \sin\theta = 9 . 8 + 0 . 2 (1 + \sin\theta)\]
\[3 . 5 - 0 . 98 \sin\theta = 1 . 96 + 1 . 96 \sin\theta\]
\[\sin\theta = \frac{1}{2}\]
\[ \Rightarrow \theta = {30}^o\]
∴ Angle of projection = 90° − 30° = 60°
Time of reaching the ground,
\[\Rightarrow t = \sqrt{\frac{2h}{g}} = \sqrt{\frac{2 \times (0 . 2 + 0 . 2\sin {30}^o )}{9 . 8}}\]
\[ \Rightarrow t = 0 . 247 s\]
Distance travelled in the horizontal direction is given by,
S = v cos θ × t
S = gr sin θ cos θ×t
S = 9.8 × 2 × 1232 × 0.247
S = 0.196 m
Total distance = (0.2 − 0.2 cos 30° + 0.196)
= 0.22 m
APPEARS IN
संबंधित प्रश्न
Consider the situation of the previous problem. Take "the table plus the ball" as the system. friction between the table and the ball is then an internal force. As the ball slows down, the momentum of the system decreases. Which external force is responsible for this change in the momentum?
Consider the following two statements:
(A) Linear momentum of a system of particles is zero.
(B) Kinetic energy of a system of particles is zero.
A nucleus moving with a velocity \[\vec{v}\] emits an α-particle. Let the velocities of the α-particle and the remaining nucleus be v1 and v2 and their masses be m1 and m2.
A shell is fired from a cannon with a velocity V at an angle θ with the horizontal direction. At the highest point in its path, it explodes into two pieces of equal masses. One of the pieces retraces its path to the cannon. The speed of the other piece immediately after the explosion is
In an elastic collision
(a) the kinetic energy remains constant
(b) the linear momentum remains constant
(c) the final kinetic energy is equal to the initial kinetic energy
(d) the final linear momentum is equal to the initial linear momentum.
A ball hits a floor and rebounds after an inelastic collision. In this case
(a) the momentum of the ball just after the collision is same as that just before the collision
(b) the mechanical energy of the ball remains the same during the collision
(c) the total momentum of the ball and the earth is conserved
(d) the total energy of the ball and the earth remains the same
A man of mass 50 kg starts moving on the earth and acquires a speed 1.8 m/s. With what speed does the earth recoil? Mass of earth = 6 × 1024 kg.
A neutron initially at rest, decays into a proton, an electron, and an antineutrino. The ejected electron has a momentum of 1.4 × 10−26 kg-m/s and the antineutrino 6.4 × 10−27kg-m/s.
Find the recoil speed of the proton
(a) if the electron and the antineutrino are ejected along the same direction and
(b) if they are ejected along perpendicular directions. Mass of the proton = 1.67 × 10−27 kg.
A gun is mounted on a railroad car. The mass of the car, the gun, the shells and the operator is 50 m where m is the mass of one shell. If the velocity of the shell with respect to the gun (in its state before firing) is 200 m/s, what is the recoil speed of the car after the second shot? Neglect friction.
A block of mass 200 g is suspended through a vertical spring. The spring is stretched by 1.0 cm when the block is in equilibrium. A particle of mass 120 g is dropped on the block from a height of 45 cm. The particle sticks to the block after the impact. Find the maximum extension of the spring. Take g = 10 m/s2.
A bullet of mass 25 g is fired horizontally into a ballistic pendulum of mass 5.0 kg and gets embedded in it. If the centre of the pendulum rises by a distance of 10 cm, find the speed of the bullet.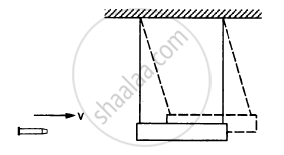
A bullet of mass 20 g moving horizontally at a speed of 300 m/s is fired into a wooden block of mass 500 g suspended by a long string. The bullet crosses the block and emerges on the other side. If the centre of mass of the block rises through a height of 20.0 cm, find the speed of the bullet as it emerges from the block.
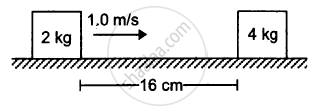
The friction coefficient between the horizontal surface and each of the block shown in figure is 0.20. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest. Take g = 10 m/s2.
A small block of superdense material has a mass of 3 × 1024kg. It is situated at a height h (much smaller than the earth's radius) from where it falls on the earth's surface. Find its speed when its height from the earth's surface has reduce to to h/2. The mass of the earth is 6 × 1024kg.
Suppose the particle of the previous problem has a mass m and a speed \[\nu\] before the collision and it sticks to the rod after the collision. The rod has a mass M. (a) Find the velocity of the centre of mass C of the system constituting "the rod plus the particle". (b) Find the velocity of the particle with respect to C before the collision. (c) Find the velocity of the rod with respect to C before the collision. (d) Find the angular momentum of the particle and of the rod about the centre of mass C before the collision. (e) Find the moment of inertia of the system about the vertical axis through the centre of mass C after the collision. (f) Find the velocity of the centre of mass C and the angular velocity of the system about the centre of mass after the collision.
A metre stick is held vertically with one end on a rough horizontal floor. It is gently allowed to fall on the floor. Assuming that the end at the floor does not slip, find the angular speed of the rod when it hits the floor.
A thin spherical shell of radius R lying on a rough horizontal surface is hit sharply and horizontally by a cue. Where should it be hit so that the shell does not slip on the surface?
