Advertisements
Advertisements
प्रश्न
The track shown is figure is frictionless. The block B of mass 2m is lying at rest and the block A or mass m is pushed along the track with some speed. The collision between Aand B is perfectly elastic. With what velocity should the block A be started to get the sleeping man awakened?

उत्तर
Given:
Mass of the block, A = m
Mass of the block, B = 2m
Let the initial velocity of block A be u1 and the final velocity of block A,when it reaches the block B be v1.
Using the work-energy theorem for block A, we can write:
Gain in kinetic energy = Loss in potential energy
`∴ (1/2)mv_1^2 - (1/2) m u_1^2 = mgh`
`Rightarrow v_1^2 - u_1^2 = 2gh`
`Rightarrow v_1= sqrt(2gh + u_1^2)` .......(1)
Let the block B just manages to reach the man's head.
i.e. the velocity of block B is zero at that point.
Again, applying the work-energy theorem for block B, we get:
`(1/2) xx 2m xx (0)^2 - (1/2 ) xx 2m xx v^2 = mgh`
`Rightarrow v = sqrt(2 gh)`
\[\text{ Therefore, before the collision }: \]
\[ \text{ Velocity of A, u}_A = v_1 \]
\[ \text{ Velocity of B, u}_B = 0\]
\[\text{ After the collision: }\]
\[ \text{ Velocity of A, v}_A = v (\text{ say })\]
\[\text{ Velocity of B, v}_B = \sqrt{2gh}\]
As the collision is elastic, K.E. and momentum are conserved.
\[m v_1 + 2m \times 0 = mv + 2m\sqrt{2gh}\]
\[ \Rightarrow v_1 - v = 2\sqrt{2gh} . . . \left( 2 \right)\]
\[\Rightarrow \left( \frac{1}{2} \right)m v_1^2 + \left( \frac{1}{2} \right)2m \times (0 )^2 = \left( \frac{1}{2} \right)m v^2 + \left( \frac{1}{2} \right)2m \left( \sqrt{2gh} \right)^2 \]
\[ \Rightarrow v_1^2 - v^2 = 2 \times \sqrt{2gh} \times \sqrt{2gh} . . . \left( 3 \right)\]
\[\text{ Dividing equation (3) by equation (2), we get: } \]
\[ v_1 + v = \sqrt{2gh} . . . \left( 4 \right)\]
\[\text{ Adding the equations } \left( 4 \right) \text{ and } \left( 2 \right), \text{ we get: } \]
\[2 v_1 = 3\sqrt{2gh}\]
\[\text{ Now using equation } \left( 1 \right) \text{ to substitue the value of v}_1 , \text{ we get: }\]
\[\sqrt{2gh + u^2} = \left( \frac{3}{2} \right)\sqrt{2gh}\]
\[ \Rightarrow 2gh + u^2 = \left( \frac{9}{4} \right)(2gh)\]
\[ \Rightarrow u = \sqrt{2 . 5 gh}\]
Block a should be started with a minimum velocity of \[\sqrt{2 . 5gh}\]
to get the sleeping man awakened.
APPEARS IN
संबंधित प्रश्न
A van is standing on a frictionless portion of a horizontal road. To start the engine, the vehicle must be set in motion in the forward direction. How can be persons sitting inside the van do it without coming out and pushing from behind?
Consider the following two statements:
(A) Linear momentum of a system of particles is zero.
(B) Kinetic energy of a system of particles is zero.
A bullet hits a block kept at rest on a smooth horizontal surface and gets embedded into it. Which of the following does not change?
Internal forces can change
The quantities remaining constant in a collisions are
A block moving in air breaks in two parts and the parts separate
(a) the total momentum must be conserved
(b) the total kinetic energy must be conserved
(c) the total momentum must change
(d) the total kinetic energy must change
In an elastic collision
(a) the kinetic energy remains constant
(b) the linear momentum remains constant
(c) the final kinetic energy is equal to the initial kinetic energy
(d) the final linear momentum is equal to the initial linear momentum.
A ball of mass m moving at a speed v makes a head-on collision with an identical ball at rest. The kinetic energy of the balls after the collision is three fourths of the original. Find the coefficient of restitution.
Two friends A and B (each weighing 40 kg) are sitting on a frictionless platform some distance d apart. A rolls a ball of mass 4 kg on the platform towards B which B catches. Then B rolls the ball towards A and A catches it. The ball keeps on moving back and forth between A and B. The ball has a fixed speed of 5 m/s on the platform. (a) Find the speed of A after he catches the ball for the first time. (c) Find the speeds of A and Bafter the all has made 5 round trips and is held by A. (d) How many times can A roll the ball? (e) Where is the centre of mass of the system "A + B + ball" at the end of the nth trip?
A bullet of mass 20 g moving horizontally at a speed of 300 m/s is fired into a wooden block of mass 500 g suspended by a long string. The bullet crosses the block and emerges on the other side. If the centre of mass of the block rises through a height of 20.0 cm, find the speed of the bullet as it emerges from the block.
Two blocks of masses m1 and m2 are connected by a spring of spring constant k (See figure). The block of mass m2 is given a sharp impulse so that it acquires a velocity v0 towards right. Find (a) the velocity of the centre of mass, (b) the maximum elongation that the spring will suffer.
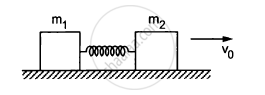
A bullet of mass 10 g moving horizontally at a speed of 50√7 m/s strikes a block of mass 490 g kept on a frictionless track as shown in figure. The bullet remains inside the block and the system proceeds towards the semicircular track of radius 0.2 m. Where will the block strike the horizontal part after leaving the semicircular track?
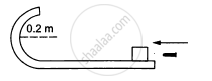
The blocks shown in figure have equal masses. The surface of A is smooth but that of Bhas a friction coefficient of 0.10 with the floor. Block A is moving at a speed of 10 m/s towards B which is kept at rest. Find the distance travelled by B if (a) the collision is perfectly elastic and (b) the collision is perfectly inelastic.
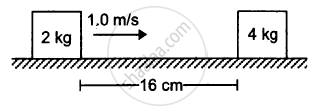
The friction coefficient between the horizontal surface and each of the block shown in figure is 0.20. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest. Take g = 10 m/s2.
A small block of superdense material has a mass of 3 × 1024kg. It is situated at a height h (much smaller than the earth's radius) from where it falls on the earth's surface. Find its speed when its height from the earth's surface has reduce to to h/2. The mass of the earth is 6 × 1024kg.
A metre stick is held vertically with one end on a rough horizontal floor. It is gently allowed to fall on the floor. Assuming that the end at the floor does not slip, find the angular speed of the rod when it hits the floor.
A small disc is set rolling with a speed \[\nu\] on the horizontal part of the track of the previous problem from right to left. To what height will it climb up the curved part?
The following figure shows a rough track, a portion of which is in the form of a cylinder of radius R. With what minimum linear speed should a sphere of radius r be set rolling on the horizontal part so that it completely goes round the circle on the cylindrical part.

A thin spherical shell of radius R lying on a rough horizontal surface is hit sharply and horizontally by a cue. Where should it be hit so that the shell does not slip on the surface?
