Advertisements
Advertisements
प्रश्न
A converging lens of focal length 12 cm and a diverging mirror of focal length 7.5 cm are placed 5.0 cm apart with their principal axes coinciding. Where should an object be placed so that its image falls on itself?
उत्तर
Let the object be placed at a distance x cm from the lens (away from the mirror).
For the convex lens (1st refraction) u = − x, f = − 12 cm
From the lens formula:
\[\frac{1}{v} - \frac{1}{u} = \frac{1}{f}\Rightarrow\frac{1}{v}=\frac{1}{( - 12)}+\frac{1}{( - x)}\Rightarrow v=-\left( \frac{12x}{x + 12} \right)\]
Thus, the virtual image due to the first refraction lies on the same side as that of object A'B'.
This image becomes the object for the convex mirror,
For the mirror,
\[u = - \left( 5 + \frac{12x}{x + 12} \right)\]
\[ = - \left( \frac{17x + 60}{x + 12} \right)\]
\[f = - 7 . 5 \text{ cm }\]
From mirror equation,
\[\frac{1}{v} + \frac{1}{u} = \frac{1}{f}\]
\[ \Rightarrow \frac{1}{v} = \frac{1}{- 7 . 5} + \frac{x + 12}{17x + 60}\]
\[ \Rightarrow \frac{1}{v} = \frac{17x + 60 - 7 . 5}{7 . 5(17x + 60)}\]
\[\Rightarrow v = \frac{7 . 5(17x + 60)}{52 . 5 - 127 . 5x}\]
\[ \Rightarrow v = \frac{250(x + 4)}{15x - 100}\]
\[ \Rightarrow v = \frac{50(x + 4)}{(3x - 20)}\]
Thus, this image is formed towards the left of the mirror.
Again for second refraction in concave lens,
\[u = - \left[ \frac{5 - 50(x + 4)}{3x - 20} \right]\]
(assuming that the image of mirror formed between the lens and mirror is 3x − 20),
v = + x (since, the final image is produced on the object A"B")
Using lens formula:
\[\frac{1}{v} - \frac{1}{u} = \frac{1}{f}\]
\[ \Rightarrow \frac{1}{x}+\frac{1}{\frac{\left[ 5 - 50 (x \times 4) \right]}{3x - 20}}=\frac{1}{- 20}\]
⇒ 25x2 − 1400x − 6000 = 0
⇒ x2 − 56x − 240 = 0
⇒ (x − 60) (x + 4) = 0
Thus, x = 60 m
The object should be placed at a distance 60 cm from the lens farther away from the mirror, so that the final image is formed on itself.
APPEARS IN
संबंधित प्रश्न
A small candle, 2.5 cm in size is placed at 27 cm in front of a concave mirror of radius of curvature 36 cm. At what distance from the mirror should a screen be placed in order to obtain a sharp image? Describe the nature and size of the image. If the candle is moved closer to the mirror, how would the screen have to be moved?
A double convex lens is made of a glass of refractive index 1.55, with both faces of the same radius of curvature. Find the radius of curvature required, if the focal length is 20 cm.
In image formation from spherical mirrors, only paraxial rays are considered because they
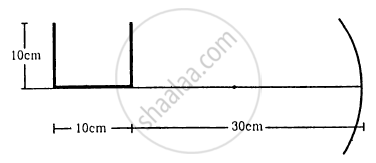
A U-shaped wire is placed before a concave mirror having radius of curvature 20 cm as shown in figure. Find the total length of the image.
A man uses a concave mirror for shaving. He keeps his face at a distance of 25 cm from the mirror and gets an image which is 1.4 times enlarged. Find the focal length of the mirror.
Consider the situation shown in figure. The elevator is going up with an acceleration of 2.00 m s−2 and the focal length of the mirror is 12.0 cm. All the surfaces are smooth and the pulley is light. The mass-pulley system is released from rest (with respect to the elevator) at t = 0 when the distance of B from the mirror is 42.0 cm. Find the distance between the image of the block B and the mirror at t = 0.200 s. Take g = 10 m s−2.

Two thin lenses having optical powers of -10D and+ 6D are placed in contact with each other. The focal length of the combination is:
Answer the following question.
Under what conditions is the phenomenon of total internal reflection of light observed? Obtain the relation between the critical angle of incidence and the refractive index of the medium.
Answer the following question.
Three lenses of focal length +10 cm, —10 cm and +30 cm are arranged coaxially as in the figure given below. Find the position of the final image formed by the combination.

Answer the following question.
With the help of a ray diagram, obtain the relation between its focal length and radius of curvature.
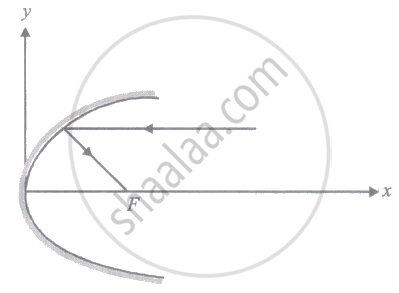
A parallel beam of light ray parallel to the x-axis is incident on a parabolic reflecting surface x = 2by2 as shown in the figure. After reflecting it passes through focal point F. What is the focal length of the reflecting surface?
The radius of curvature of the curved surface of a plano-convex lens is 20 cm. If the refractive index of the material of the lens be 1.5, it will ______.
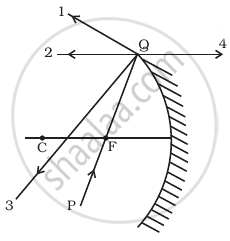
The direction of ray of light incident on a concave mirror is shown by PQ while directions in which the ray would travel after reflection is shown by four rays marked 1, 2, 3 and 4 (figure). Which of the four rays correctly shows the direction of reflected ray?
An astronomical refractive telescope has an objective of focal length 20 m and an eyepiece of focal length 2 cm.
- The length of the telescope tube is 20.02 m.
- The magnification is 1000.
- The image formed is inverted.
- An objective of a larger aperture will increase the brightness and reduce chromatic aberration of the image.
Parallel rays striking a spherical mirror far from the optic axis are focussed at a different point than are rays near the axis thereby the focus moves toward the mirror as the parallel rays move toward the outer edge of the mirror. What value of incidence angle θ produces a 2% change in the location of the focus, compared to the location for θ very close to zero?
A particle is dropped along the axis from a height 15 cm on a concave mirror of focal length 30 cm as shown in figure. The acceleration due to gravity is 10 m/s2. Find the maximum speed of image in m/s:

If an object is placed at a distance of 10 cm in front of a concave mirror of a focal length of 20 cm, the image formed will be ______.
Why does a car driver use a convex mirror as a rear-view mirror?
A lens of focal length f is divided into two equal parts and then these parts are put in a combination as shown in the figure below.
- What is the focal length of L1?
- What is the focal length of the final combination?

