Advertisements
Advertisements
प्रश्न
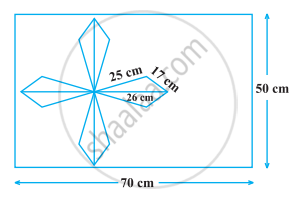
A design is made on a rectangular tile of dimensions 50 cm × 70 cm as shown in the following figure. The design shows 8 triangles, each of sides 26 cm, 17 cm and 25 cm. Find the total area of the design and the remaining area of the tile.
उत्तर
Given, the dimension of rectangular tile is 50 cm × 70 cm.
∴ Area of rectangular tile = 50 × 70 = 3500 cm2
The sides of a design of one triangle be
a = 25 cm, b = 17 cm and c = 26 cm
Now, semi-perimeter,
`s = (a + b + c)/2`
= `(25 + 17 + 26)/2`
= `68/2`
= 34
∴ Area of one triangle = `sqrt(s(s - a)(s - b)(s - c))` ...[By Heron’s formula]
= `sqrt(34 xx 9 xx 17 xx 8)`
= `sqrt(17 xx 2 xx 3 xx 3 xx 17 xx 2 xx 2 xx 2)`
= 17 × 3 × 2 × 2
= 204 cm2
∴ Total area of eight triangles = 204 × 8 = 1632 cm2
Now, area of the design = Total area of eight triangles
= 1632 cm2
Also, remaining area of the tile = Area of the rectangle – Area of the design
= 3500 – 1632
= 1868 cm2
Hence, the total area of the design is 1632 cm2 and the remaining area of the tile is 1868 cm2.
APPEARS IN
संबंधित प्रश्न
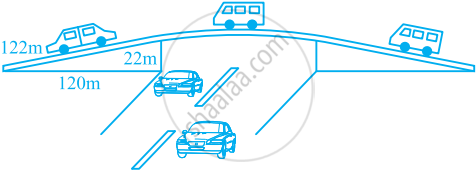
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 122m, 22m, and 120m (see the given figure). The advertisements yield an earning of ₹ 5000 per m2 per year. A company hired one of its walls for 3 months. How much rent did it pay?
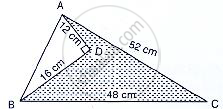
Find the area of the shaded region in the given figure.
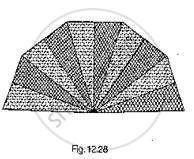
A hand fan is made by stitching lo equal size triangular strips of two different types of paper as shown in Fig. 12.28. The dimensions of equal strips are 25 cm, 25 cm and 14 cm. Find the area of each type of paper needed to make the hand fan.
Find the areas of the given plot. (All measures are in metres.)
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
If (5, 7), (3, p) and (6, 6) are collinear, then the value of p is
The sides of the triangular ground are 22 m, 120 m and 122 m. Find the area and cost of levelling the ground at the rate of ₹ 20 per m2
The perimeter of a triangular plot is 600 m. If the sides are in the ratio 5 : 12 : 13, then find the area of the plot
The perimeter of a triangle is 50 cm. One side of a triangle is 4 cm longer than the smaller side and the third side is 6 cm less than twice the smaller side. Find the area of the triangle.
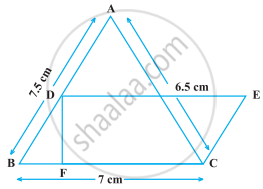
In the following figure, ∆ABC has sides AB = 7.5 cm, AC = 6.5 cm and BC = 7 cm. On base BC a parallelogram DBCE of same area as that of ∆ABC is constructed. Find the height DF of the parallelogram.