Advertisements
Advertisements
प्रश्न
A field in the form of a parallelogram has sides 60 m and 40 m and one of its diagonals is 80 m long. Find the area of the parallelogram.
उत्तर
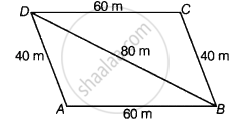
Let ABCD be a parallelogram field with sides AB = CD = 60 m, BC = DA = 40 m and diagonal BD = 80 m.
Area of parallelogram ABCD = 2(Area of ΔABD) ...(i)
In ΔABD,
Semi-perimeter of a triangle ΔABD,
`s = (a + b + c)/2`
= `(AB + BD + DA)/2`
= `(60 + 80 + 40)/2`
= `180/2`
= 90 m
∴ Area of ΔABD = `sqrt(s(s - a)(s - b)(s - c))` ...[By Heron’s formula]
= `sqrt(90(90 - 60)(90 - 80)(90 - 40))`
= `sqrt(90 xx 30 xx 10 xx 50)`
= `100 xx 3sqrt(15)`
= `300sqrt(15) m^2`
From equation (i),
Area of parallelogram ABCD = `2 xx 300sqrt(15) = 600sqrt(15) m^2`
Hence, the area of the parallelogram is `600sqrt(15) m^2`.
APPEARS IN
संबंधित प्रश्न
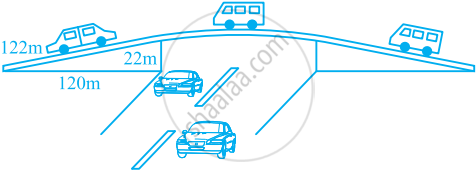
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 122m, 22m, and 120m (see the given figure). The advertisements yield an earning of ₹ 5000 per m2 per year. A company hired one of its walls for 3 months. How much rent did it pay?
Find the area of a triangle two sides of which are 18 cm and 10 cm and the perimeter is 42 cm.
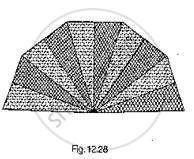
A hand fan is made by stitching lo equal size triangular strips of two different types of paper as shown in Fig. 12.28. The dimensions of equal strips are 25 cm, 25 cm and 14 cm. Find the area of each type of paper needed to make the hand fan.
Find the area of the triangle formed by the points
(1, – 1), (– 4, 6) and (– 3, – 5)
Find the area of the triangle formed by the points
(–10, –4), (–8, –1) and (–3, –5)
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
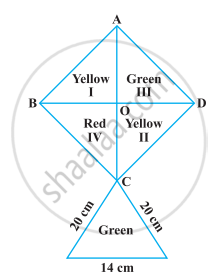
Find the area of triangle FED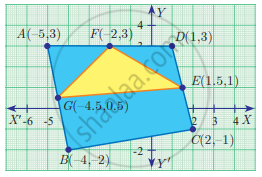
Find the area of a triangle formed by the lines 3x + y – 2 = 0, 5x + 2y – 3 = 0 and 2x – y – 3 = 0
An isosceles right triangle has area 8 cm2. The length of its hypotenuse is ______.
How much paper of each shade is needed to make a kite given in the following figure, in which ABCD is a square with diagonal 44 cm.