Advertisements
Advertisements
प्रश्न
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140º, then ∠BAC is equal to ______.
विकल्प
80º
50º
40º
30º
उत्तर
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140º, then ∠BAC is equal to 50º.
Explanation:
Given, ABCD is a cyclic quadrilateral and ∠ADC = 140°.
We know that, sum of the opposite angles in a cyclic quadrilateral is 180°.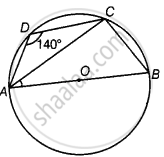
∠ADC + ∠ABC = 180°
⇒ 140° + ∠ABC = 180°
⇒ ∠ABC = 180° – 140°
∴ ∠ABC = 40°
Since, ∠ACB is an angle in a semi-circle.
∴ ∠ACB = 90°
In ΔABC, ∠BAC + ∠ACB + ∠ABC = 180° ...[By angle sum property of a triangle]
⇒ ∠BAC + 90° + 40° = 180°
⇒ ∠BAC = 180° – 130° = 50°
APPEARS IN
संबंधित प्रश्न
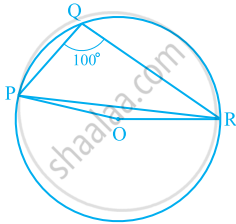
In the given figure, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.
If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
Prove that a cyclic parallelogram is a rectangle.
In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.
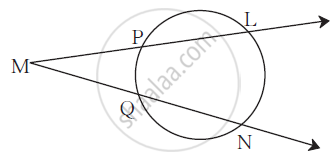
In the figure m(arc LN) = 110°,
m(arc PQ) = 50° then complete the following activity to find ∠LMN.
∠ LMN = `1/2` [m(arc LN) - _______]
∴ ∠ LMN = `1/2` [_________ - 50°]
∴ ∠ LMN = `1/2` × _________
∴ ∠ LMN = __________
Prove that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent.
ABCD is a cyclic quadrilateral in which BA and CD when produced meet in E and EA = ED. Prove that EB = EC.
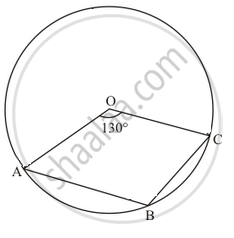
In the given figure, O is the centre of the circle such that ∠AOC = 130°, then ∠ABC =
If P, Q and R are the mid-points of the sides BC, CA and AB of a triangle and AD is the perpendicular from A on BC, prove that P, Q, R and D are concyclic.
If bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
