Advertisements
Advertisements
प्रश्न
In the following figure, BC is a diameter of the circle and ∠BAO = 60º. Then ∠ADC is equal to ______.
विकल्प
30º
45º
60º
120º
Advertisements
उत्तर
In the following figure, BC is a diameter of the circle and ∠BAO = 60º. Then ∠ADC is equal to 60º.
Explanation:
Given: BC is a diameter of the circle and ∠BAO = 60º.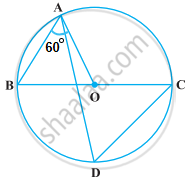
Now, in triangle OAB,
OA = OB ...[Radii of the same circle]
So, ∠ABO = ∠BAO ...[Angle opposite to equal sides are equal]
∠ABO = ∠BAO = 60º ...[Given]
Now, ∠ADC = ∠ABC = 60º ...[∠ADC and ∠ABC are angles in the same segment of a circle are equal]
Therefore, ∠ADC = 60º.
APPEARS IN
संबंधित प्रश्न
ABCD is a quadrilateral such that ∠D = 90°. A circle (O, r) touches the sides AB, BC, CD and DA at P,Q,R and If BC = 38 cm, CD = 25 cm and BP = 27 cm, find r.
In fig., O is the centre of the circle, PA and PB are tangent segments. Show that the quadrilateral AOBP is cyclic.
In Fig below, PQ is tangent at point R of the circle with center O. If ∠TRQ = 30°. Find
∠PRS.
In the given figure, a circle with center O, is inscribed in a quadrilateral ABCD such that it touches the side BC, AB, AD and CD at points P, Q, R and S respectively. If AB = 29cm, AD = 23cm, ∠B = 90° and DS=5cm then find the radius of the circle.
If AB, BC and CD are equal chords of a circle with O as centre and AD diameter, than ∠AOB =
Equal circles with centres O and O' touch each other at X. OO' produced to meet a circle with centre O', at A. AC is a tangent to the circle whose centre is O. O'D is perpendicular to AC. Find the value of\[\frac{DO'}{CO}\]
The circle which passes through all the vertices of a triangle is called ______.
Use the figure given below to fill in the blank:
Tangent to a circle is _______.
Let s denote the semi-perimeter of a triangle ABC in which BC = a, CA = b, AB = c. If a circle touches the sides BC, CA, AB at D, E, F, respectively, prove that BD = s – b.
If AOB is a diameter of a circle and C is a point on the circle, then AC2 + BC2 = AB2.
