Advertisements
Advertisements
प्रश्न
Prove that a cyclic parallelogram is a rectangle.
उत्तर
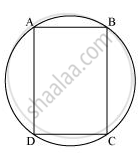
Let ABCD be a cyclic parallelogram.
∠A + ∠C = 180° (Opposite angles of a cyclic quadrilateral) ...(1)
We know that opposite angles of a parallelogram are equal.
∴ ∠A = ∠C and ∠B = ∠D
From equation (1),
∠A + ∠C = 180°
⇒ ∠A + ∠A = 180°
⇒ 2 ∠A = 180°
⇒ ∠A = 90°
Parallelogram ABCD has one of its interior angles, which is 90°. Therefore, it is a rectangle.
APPEARS IN
संबंधित प्रश्न
Prove that "Opposite angles of a cyclic quadrilateral are supplementary".
In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.
Two chords AB and CD of lengths 5 cm 11cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of the triangle DEF are `90^@-1/2A, 90^@-1/2B" and "90^@-1/2C`
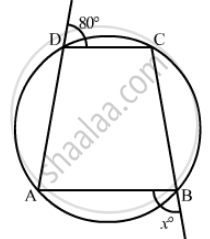
In the given figure, ABCD is a cyclic quadrilateral. Find the value of x.
ABCD is a cyclic quadrilateral such that ∠ADB = 30° and ∠DCA = 80°, then ∠DAB =
ABCD is a cyclic quadrilateral. M (arc ABC) = 230°. Find ∠ABC, ∠CDA, and ∠CBE.
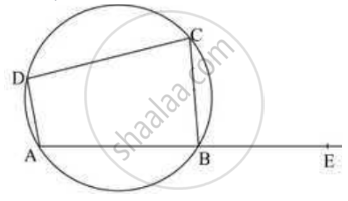
In the figure, ▢ABCD is a cyclic quadrilateral. If m(arc ABC) = 230°, then find ∠ABC, ∠CDA, ∠CBE.

ABCD is a cyclic quadrilateral such that ∠A = 90°, ∠B = 70°, ∠C = 95° and ∠D = 105°.
If non-parallel sides of a trapezium are equal, prove that it is cyclic.
