Advertisements
Advertisements
प्रश्न
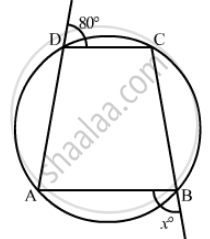
In the given figure, ABCD is a cyclic quadrilateral. Find the value of x.
उत्तर
Here, ABCD is a cyclic quadrilateral, we need to find x.
In cyclic quadrilateral the sum of opposite angles is equal to 180°.
Therefore,
\[\angle ADC + \angle ABC = 180°\]
\[ \Rightarrow 180° - 80°+ 180°- x = 180° \]
\[ \Rightarrow x = 100° \]
Hence, the value of x is 100°.
APPEARS IN
संबंधित प्रश्न
If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
Prove that the circle drawn with any side of a rhombus as diameter passes through the point of intersection of its diagonals.
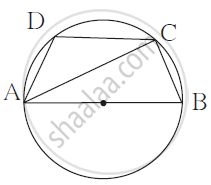
In the figure, `square`ABCD is a cyclic quadrilateral. Seg AB is a diameter. If ∠ ADC = 120˚, complete the following activity to find measure of ∠ BAC.
`square` ABCD is a cyclic quadrilateral.
∴ ∠ ADC + ∠ ABC = 180°
∴ 120˚ + ∠ ABC = 180°
∴ ∠ ABC = ______
But ∠ ACB = ______ .......(angle in semicircle)
In Δ ABC,
∠ BAC + ∠ ACB + ∠ ABC = 180°
∴ ∠BAC + ______ = 180°
∴ ∠ BAC = ______
ABCD is a cyclic quadrilateral in ∠DBC = 80° and ∠BAC = 40°. Find ∠BCD.
Prove that the circles described on the four sides of a rhombus as diameters, pass through the point of intersection of its diagonals.
ABCD is a cyclic trapezium with AD || BC. If ∠B = 70°, determine other three angles of the trapezium.
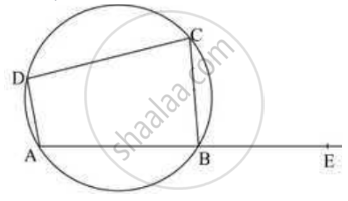
ABCD is a cyclic quadrilateral. M (arc ABC) = 230°. Find ∠ABC, ∠CDA, and ∠CBE.
In a cyclic quadrilaterals ABCD, ∠A = 4x, ∠C = 2x the value of x is
If P, Q and R are the mid-points of the sides BC, CA and AB of a triangle and AD is the perpendicular from A on BC, prove that P, Q, R and D are concyclic.
ABCD is a parallelogram. A circle through A, B is so drawn that it intersects AD at P and BC at Q. Prove that P, Q, C and D are concyclic.
