Advertisements
Advertisements
प्रश्न
Prove that the circles described on the four sides of a rhombus as diameters, pass through the point of intersection of its diagonals.
Advertisements
उत्तर
Here, ABCD is a rhombus; we have to prove the four circles described on the four sides of any rhombusABCD pass through the point of intersection of its diagonals AC and BD.
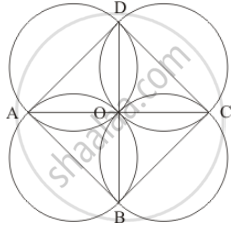
Let the diagonals AC and BD intersect at O.
We know that the diagonals of a rhombus intersect at right angle.
Therefore,
`angle AOB = `90°
`angleBOC = `90°
`angle COD ` = 90°
`angle AOD ` = 90°
Now, `angle AOB ` = 90 means that circle described on AB as diameter passes through O.
Similarly the remaining three circles with BC, CD and AD as their diameter will also pass through O.
Hence, all the circles with described on the four sides of any rhombus ABCD pass through the point of intersection of its diagonals AC and BD.
APPEARS IN
संबंधित प्रश्न
Prove that ‘Opposite angles of a cyclic quadrilateral are supplementary’.
ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD.
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
Circles are described on the sides of a triangle as diameters. Prove that the circles on any two sides intersect each other on the third side (or third side produced).
ABCD is a cyclic trapezium with AD || BC. If ∠B = 70°, determine other three angles of the trapezium.
Prove that the centre of the circle circumscribing the cyclic rectangle ABCD is the point of intersection of its diagonals.
In the given figure, O is the centre of the circle such that ∠AOC = 130°, then ∠ABC =
Find all the angles of the given cyclic quadrilateral ABCD in the figure.
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140º, then ∠BAC is equal to ______.
If a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are also equal.
