Advertisements
Advertisements
प्रश्न
If the two sides of a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are equal.
उत्तर
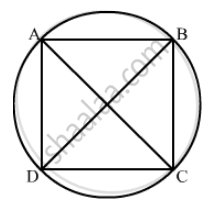
To prove: AC = BD
Proof: We know that equal chords subtend equal at the centre of circle and the angle subtended by a chord at the centre is twice the angle subtended by it at remaining part of the circle.
\[\angle AOD = \angle BOC \left( \text{ O is the centre of the circle } \right)\]
\[\angle AOD = 2\angle ACD \]
\[\text{ and } \angle BOC = 2\angle BDC\]
\[\text{ Since, } \angle AOD = \angle BOC\]
\[ \Rightarrow \angle ACD = \angle BDC . . . . . \left( 1 \right) \]
\[\angle ACB = \angle ADB . . . . . \left( 2 \right) \left( \text{ Angle in the same segment are equal } \right)\]
\[\text{ Adding } \left( 1 \right) \text{ and } \left( 2 \right)\]
\[\angle BCD = \angle ADC . . . . . \left( 3 \right)\]
\[\text{ In } \bigtriangleup ACD \text{ and } \bigtriangleup BDC\]
\[CD = CD \left( \text{ common } \right)\]
\[\angle BCD = \angle ADC \left[ \text{ Using } \left( 3 \right) \right]\]
\[AD = BC \left( given \right)\]
\[\text{ Hence } , \bigtriangleup ACD \cong BDC \left( \text{ SAS congruency criterion } \right)\]
\[ \therefore AC = BD \left( \text{ cpct } \right)\]
Hence Proved
APPEARS IN
संबंधित प्रश्न
Let the vertex of an angle ABC be located outside a circle and let the sides of the angle intersect equal chords AD and CE with the circle. Prove that ∠ABC is equal to half the difference of the angles subtended by the chords AC and DE at the centre.
ABCD is a parallelogram. The circle through A, B and C intersect CD (produced if necessary) at E. Prove that AE = AD.
Two chords AB and CD of lengths 5 cm 11cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
ABCD is a cyclic quadrilateral in BC || AD, ∠ADC = 110° and ∠BAC = 50°. Find ∠DAC.
ABCD is a cyclic quadrilateral in ∠DBC = 80° and ∠BAC = 40°. Find ∠BCD.
Prove that the circles described on the four sides of a rhombus as diameters, pass through the point of intersection of its diagonals.
In the figure, ▢ABCD is a cyclic quadrilateral. If m(arc ABC) = 230°, then find ∠ABC, ∠CDA, ∠CBE.

In the following figure, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ACD + ∠BED.
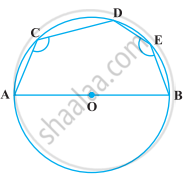
ABCD is a parallelogram. A circle through A, B is so drawn that it intersects AD at P and BC at Q. Prove that P, Q, C and D are concyclic.
The three angles of a quadrilateral are 100°, 60°, 70°. Find the fourth angle.
