Advertisements
Advertisements
प्रश्न
ABCD is a parallelogram. The circle through A, B and C intersect CD (produced if necessary) at E. Prove that AE = AD.
उत्तर
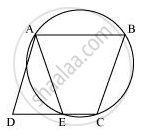
It can be observed that ABCE is a cyclic quadrilateral and in a cyclic quadrilateral, the sum of the opposite angles is 180°.
∠AEC + ∠CBA = 180°
∠AEC + ∠AED = 180° (Linear pair)
∠AED = ∠CBA ... (1)
For a parallelogram, opposite angles are equal.
∠ADE = ∠CBA ... (2)
From (1) and (2),
∠AED = ∠ADE
AD = AE (Angles opposite to equal sides of a triangle)
APPEARS IN
संबंधित प्रश्न
A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
Prove that a cyclic parallelogram is a rectangle.
Prove that the line of centres of two intersecting circles subtends equal angles at the two points of intersection.
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
Two chords AB and CD of lengths 5 cm 11cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
In the given figure, ∠BAD = 78°, ∠DCF = x° and ∠DEF = y°. Find the values of x and y.

ABCD is a cyclic quadrilateral in ∠BCD = 100° and ∠ABD = 70° find ∠ADB.
Prove that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent.
If a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral so formed is cyclic.
