Advertisements
Advertisements
प्रश्न
Prove that "Opposite angles of a cyclic quadrilateral are supplementary".
उत्तर
Given:- □ABCD is a cyclic quadrilateral.
To prove:- ∠BAD + ∠BCD = 180º and ∠ABC + ∠ADC = 180º
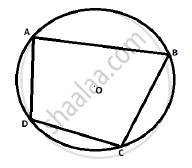
Proof:- Arc BCD is intercepted by the inscribed ∠BAD.
∠BAD = `1/2` m(arc BCD) ..........(i) [Inscribed angle theorem]
Arc BAD is intercepted by the inscribed ∠BCD.
∴ ∠BCD = `1/2` m(arc DAB) ..........(ii) [Inscribed angle theorem]
From (1) and (2) we get
∠BAD + ∠BCD = `1/2` [m(arc BCD) + m(arc DAB)]
∴ (∠BAD + ∠BCD) = `1/2 xx 360^circ` .....[Completed circle]
= 180°
Again, as the sum of the measures of angles of a quadrilateral is 360°
∴ ∠ADC + ∠ABC = 360° – [∠BAD + ∠BCD]
= 360° – 180°
= 180°
Hence the opposite angles of a cyclic quadrilateral are supplementary.
APPEARS IN
संबंधित प्रश्न
A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively (see the given figure). Prove that ∠ACP = ∠QCD.

ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD.
Prove that a cyclic parallelogram is a rectangle.
Let the vertex of an angle ABC be located outside a circle and let the sides of the angle intersect equal chords AD and CE with the circle. Prove that ∠ABC is equal to half the difference of the angles subtended by the chords AC and DE at the centre.
In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.
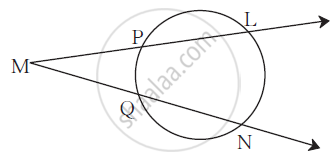
In the figure m(arc LN) = 110°,
m(arc PQ) = 50° then complete the following activity to find ∠LMN.
∠ LMN = `1/2` [m(arc LN) - _______]
∴ ∠ LMN = `1/2` [_________ - 50°]
∴ ∠ LMN = `1/2` × _________
∴ ∠ LMN = __________
In the given figure, ∠BAD = 78°, ∠DCF = x° and ∠DEF = y°. Find the values of x and y.

In the given figure, ABCD is a cyclic quadrilateral. Find the value of x.
ABCD is a cyclic quadrilateral in ∠BCD = 100° and ∠ABD = 70° find ∠ADB.
Prove that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent.
PQRS is a cyclic quadrilateral such that PR is a diameter of the circle. If ∠QPR = 67° and ∠SPR = 72°, then ∠QRS =
In the given figure, O is the centre of the circle such that ∠AOC = 130°, then ∠ABC =
ABCD is a cyclic quadrilateral. M (arc ABC) = 230°. Find ∠ABC, ∠CDA, and ∠CBE.
Find all the angles of the given cyclic quadrilateral ABCD in the figure.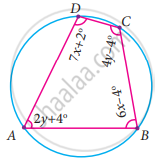
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140º, then ∠BAC is equal to ______.
If a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral so formed is cyclic.
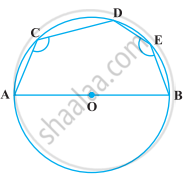
In the following figure, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ACD + ∠BED.
If P, Q and R are the mid-points of the sides BC, CA and AB of a triangle and AD is the perpendicular from A on BC, prove that P, Q, R and D are concyclic.
