Advertisements
Advertisements
प्रश्न
If bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
उत्तर
Given: ABCD is a cyclic quadrilateral.
DP and QB are the bisectors of ∠D and ∠B, respectively.
To prove: PQ is the diameter of a circle.
Construction: Join QD and QC.

Proof: Since, ABCD is a cyclic quadrilateral.
∴ ∠CDA + ∠CBA = 180° ...[Sum of opposite angles of cyclic quadrilateral is 180°]
On dividing both sides by 2, we get
`1/2 ∠CDA + 1/2 ∠CBA = 1/2 xx 180^circ = 90^circ`
⇒ ∠1 + ∠2 = 90° ...(i) `[∠1 = 1/2 ∠CDA "and" ∠2 = 1/2 ∠CBA]`
But ∠2 = ∠3 [Angles in the same segment QC are equal] ...(ii)
∠1 + ∠3 = 90°
From equations (i) and (ii),
∠PDQ = 90°
Hence, PQ is a diameter of a circle, because diameter of the circle.
Subtends a right angle at the circumference.
APPEARS IN
संबंधित प्रश्न
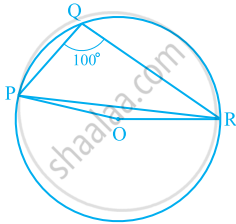
In the given figure, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.
ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠DBC = 70°, ∠BAC is 30°, find ∠BCD. Further, if AB = BC, find ∠ECD.
ABCD is a parallelogram. The circle through A, B and C intersect CD (produced if necessary) at E. Prove that AE = AD.
In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.
The lengths of two parallel chords of a circle are 6 cm and 8 cm. If the smaller chord is at distance 4 cm from the centre, what is the distance of the other chord from the centre?
In the given figure, ∠BAD = 78°, ∠DCF = x° and ∠DEF = y°. Find the values of x and y.

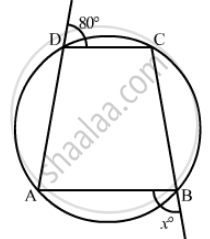
In the given figure, ABCD is a cyclic quadrilateral. Find the value of x.
ABCD is a cyclic quadrilateral in BC || AD, ∠ADC = 110° and ∠BAC = 50°. Find ∠DAC.
ABCD is a cyclic quadrilateral in which BA and CD when produced meet in E and EA = ED. Prove that AD || BC .
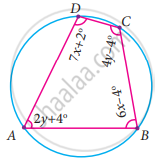
Find all the angles of the given cyclic quadrilateral ABCD in the figure.