Advertisements
Advertisements
प्रश्न
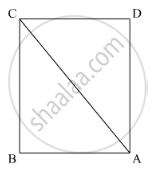
ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA (See the given figure). Prove that
- ΔABD ≅ ΔBAC
- BD = AC
- ∠ABD = ∠BAC.

उत्तर
In quadrilateral ABCD, we have
AD = BC and ∠DAB = ∠CBA
i. In ΔABD and ΔBAC,
AD = BC ...[Given]
∠DAB = ∠CBA ...[Given]
AB = BA ...[Common]
∴ ΔABD ≅ ΔBAC ...[By SAS congruency]
ii. Since ΔABD ≅ ΔBAC
BD = AC ...[By Corresponding parts of congruent triangles]
iii. Since ΔABD ≅ ΔBAC
∠ABD = ∠BAC ...[By Corresponding parts of congruent triangles]
APPEARS IN
संबंधित प्रश्न
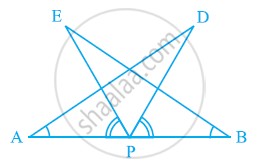
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB (See the given figure). Show that
- ΔDAP ≅ ΔEBP
- AD = BE
Which congruence criterion do you use in the following?
Given: AC = DF
AB = DE
BC = EF
So, ΔABC ≅ ΔDEF

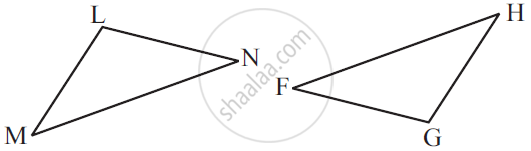
Which congruence criterion do you use in the following?
Given: ∠MLN = ∠FGH
∠NML = ∠GFH
ML = FG
So, ΔLMN ≅ ΔGFH
In the given figure, prove that:
CD + DA + AB + BC > 2AC
If the following pair of the triangle is congruent? state the condition of congruency:
In ΔABC and ΔQRP, AB = QR, ∠B = ∠R and ∠C = P.
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
Prove that: AB = BL.
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
In ΔABC, AB = AC and the bisectors of angles B and C intersect at point O.
Prove that : (i) BO = CO
(ii) AO bisects angle BAC.
In a triangle, ABC, AB = BC, AD is perpendicular to side BC and CE is perpendicular to side AB.
Prove that: AD = CE.
ABC is an isosceles triangle with AB = AC and BD and CE are its two medians. Show that BD = CE.
