Advertisements
Advertisements
प्रश्न
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
उत्तर

In ΔAOD and ΔAOB,
AD = AB ...(given)
AO = AO ...(Common)
OD = OB ...(given)
⇒ ΔAOD ≅ ΔAOB ...(by SSS congruence criterion)
⇒ ∠AOD = ∠AOB ...(c.p.c.t.) ...(i)
Similarly, ΔDOC ≅ ΔBOC
⇒ ∠DOC = ∠BOC ...(c.p.c.t.) ...(ii)
But, ∠AOB + ∠AOD + ∠COD + ∠BOC = 4 Right angles ...[ Sum of the angles at a point is 4 Right angles ]
⇒ 2∠AOD + 2∠COD = 4 Right angles ....[ Using (i) and (ii) ]
⇒ ∠AOD + ∠COD = 2 Right angles
⇒ ∠AOD + ∠COD = 180°
⇒ ∠AOD and ∠COD form a linear pair.
⇒ AO and OC are in the same straight line.
⇒ AOC is a straight line.
APPEARS IN
संबंधित प्रश्न
AD and BC are equal perpendiculars to a line segment AB (See the given figure). Show that CD bisects AB.

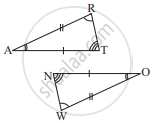
Which congruence criterion do you use in the following?
Given: ZX = RP
RQ = ZY
∠PRQ = ∠XZY
So, ΔPQR ≅ ΔXYZ

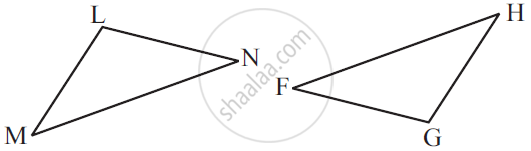
Which congruence criterion do you use in the following?
Given: ∠MLN = ∠FGH
∠NML = ∠GFH
ML = FG
So, ΔLMN ≅ ΔGFH
Which congruence criterion do you use in the following?
Given: EB = DB
AE = BC
∠A = ∠C = 90°
So, ΔABE ≅ ΔCDB

In the figure, the two triangles are congruent.
The corresponding parts are marked. We can write ΔRAT ≅ ?
In Fig. 10.92, it is given that AB = CD and AD = BC. Prove that ΔADC ≅ ΔCBA.

In the given figure, ABC is an isosceles triangle whose side AC is produced to E. Through C, CD is drawn parallel to BA. The value of x is

In ∆ABC, AB = AC. Show that the altitude AD is median also.
In the following figure, ∠A = ∠C and AB = BC.
Prove that ΔABD ≅ ΔCBE. 
In the following figure, ABC is an equilateral triangle in which QP is parallel to AC. Side AC is produced up to point R so that CR = BP.
Prove that QR bisects PC.
Hint: ( Show that ∆ QBP is equilateral
⇒ BP = PQ, but BP = CR
⇒ PQ = CR ⇒ ∆ QPM ≅ ∆ RCM ).
