Advertisements
Advertisements
प्रश्न
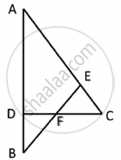
ABCD is a parallelogram in which P is the midpoint of DC and Q is a point on AC such that CQ = `1/4` AC. If PQ produced meets BC at R, prove that R is the midpoint of BC.

उत्तर
We know that the diagonals of a parallelogram bisect each other.
Therefore,
`CS=1/2AC` ................(1)
Also, it is given that` CQ=1/4 AC ` .............(2)
Dividing equation (ii) by (i), we get:
`CQ/CS=((1/4)AC)/((1/2)AC)`
Or, CQ=`1/2`CS
Hence, Q is the midpoint of CS.
Therefore, according to midpoint theorem in ΔCSD
PQ || DS
If PQ || DS, we can say that QR || SB
In Δ CSB, Q is midpoint of CS and QR ‖ SB.
Applying converse of midpoint theorem , we conclude that R is the midpoint of CB.
This completes the proof.
APPEARS IN
संबंधित प्रश्न
In the given figure, D is a point on side BC of ΔABC such that ∠ADC=∠BAC . Prove that AD is the bisector of ∠BAC.
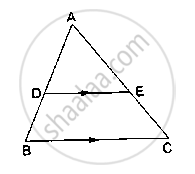
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"DB"=3/4` and AC = 15 cm, find AE
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC.
If AB = 13.3cm, AC = 11.9cm and EC = 5.1cm, find AD.
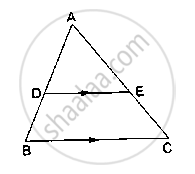
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC.
If` (AD)/(AB) = 8/15 and EC = 3.5cm`, find AE.
In the given figure, ABCD is a trapezium in which AB║DC and its diagonals intersect at O. If AO = (5x – 7), OC = (2x + 1) , BO = (7x – 5) and OD = (7x + 1), find the value of x.

Find the length of a diagonal of a rectangle whose adjacent sides are 30cm and 16cm.
In ΔABC, D is the midpoint of BC and AE⊥BC. If AC>AB, show that `AB^2= AD^2+1/4 BC^2 −BC.DE `
In triangle BMP and CNR it is given that PB= 5 cm, MP = 6cm BM = 9 cm and NR = 9cm. If ΔBMP∼ ΔCNR then find the perimeter of ΔCNR
In the given figure, DE || BC. If AD = 3 cm, AB = 7 cm and EC = 3 cm, then the length of AE is ______.
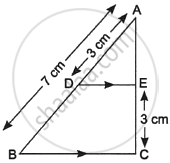
In the given figure ∠CEF = ∠CFE. F is the midpoint of DC. Prove that `(AB)/(BD) = (AE)/(FD)`