Advertisements
Advertisements
प्रश्न
Add vectors \[\vec{A} , \vec{B} \text { and } \vec{C}\] each having magnitude of 100 unit and inclined to the X-axis at angles 45°, 135° and 315° respectively.
Advertisements
उत्तर
First, we will find the components of the vector along the x-axis and y-axis. Then we will find the resultant x and y-components.
x-component of \[\vec{A} = \ A\ cos \ 45^\circ =100 \cos 45^\circ = \frac{100}{\sqrt{2}} \text { unit }\]
x-component of \[\vec{B} = \vec{B} \cos 135^\circ = - \frac{100}{\sqrt{2}}\]
x-component of \[\vec{C}\] = \[\vec{C}\] cos 315\[^\circ\]
= 100 cos 315°
\[\tan \alpha = \frac{\text { y comp}}{\text { x comp }}\]
\[ = \frac{100\sqrt{2}}{100\sqrt{2}} = 1\]
⇒ α = tan−1 (1) = 45°
∴ The magnitude of the resultant vector is 100 units and it makes an angle of 45° with the x-axis.
APPEARS IN
संबंधित प्रश्न
What are the dimensions of volume of a cube of edge a.
Suppose a quantity x can be dimensionally represented in terms of M, L and T, that is, `[ x ] = M^a L^b T^c`. The quantity mass
Choose the correct statements(s):
(a) All quantities may be represented dimensionally in terms of the base quantities.
(b) A base quantity cannot be represented dimensionally in terms of the rest of the base quantities.
(c) The dimensions of a base quantity in other base quantities is always zero.
(d) The dimension of a derived quantity is never zero in any base quantity.
Find the dimensions of linear momentum .
Find the dimensions of
(a) angular speed ω,
(b) angular acceleration α,
(c) torque τ and
(d) moment of interia I.
Some of the equations involving these quantities are \[\omega = \frac{\theta_2 - \theta_1}{t_2 - t_1}, \alpha = \frac{\omega_2 - \omega_1}{t_2 - t_1}, \tau = F . r \text{ and }I = m r^2\].
The symbols have standard meanings.
Find the dimensions of electric field E.
The relevant equations are \[F = qE, F = qvB, \text{ and }B = \frac{\mu_0 I}{2 \pi a};\]
where F is force, q is charge, v is speed, I is current, and a is distance.
Find the dimensions of magnetic field B.
The relevant equation are \[F = qE, F = qvB, \text{ and }B = \frac{\mu_0 I}{2 \pi a};\]
where F is force, q is charge, v is speed, I is current, and a is distance.
Test if the following equation is dimensionally correct:
\[v = \sqrt{\frac{P}{\rho}},\]
where v = velocity, ρ = density, P = pressure
Test if the following equation is dimensionally correct:
\[v = \frac{1}{2 \pi}\sqrt{\frac{mgl}{I}};\]
where h = height, S = surface tension, \[\rho\] = density, P = pressure, V = volume, \[\eta =\] coefficient of viscosity, v = frequency and I = moment of interia.
Is it possible to add two vectors of unequal magnitudes and get zero? Is it possible to add three vectors of equal magnitudes and get zero?
The resultant of \[\vec{A} \text { and } \vec{B}\] makes an angle α with \[\vec{A}\] and β with \[\vec{B}\],
A vector \[\vec{A}\] points vertically upward and \[\vec{B}\] points towards the north. The vector product \[\vec{A} \times \vec{B}\] is
The magnitude of the vector product of two vectors \[\left| \vec{A} \right|\] and \[\left| \vec{B} \right|\] may be
(a) greater than AB
(b) equal to AB
(c) less than AB
(d) equal to zero.
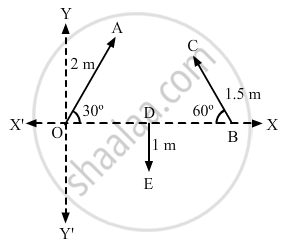
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
Let \[\vec{a} = 2 \vec{i} + 3 \vec{j} + 4 \vec{k} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j} + 5 \vec{k}\] Find the angle between them.
If \[\vec{A} , \vec{B} , \vec{C}\] are mutually perpendicular, show that \[\vec{C} \times \left( \vec{A} \times \vec{B} \right) = 0\] Is the converse true?
The electric current in a charging R−C circuit is given by i = i0 e−t/RC where i0, R and C are constant parameters of the circuit and t is time. Find the rate of change of current at (a) t = 0, (b) t = RC, (c) t = 10 RC.
Write the number of significant digits in (a) 1001, (b) 100.1, (c) 100.10, (d) 0.001001.
High speed moving particles are studied under
