Advertisements
Advertisements
प्रश्न
D(−1, 8), E(4, −2), F(−5, −3) are midpoints of sides BC, CA and AB of ∆ABC Find co-ordinates of the circumcenter of ΔABC
उत्तर
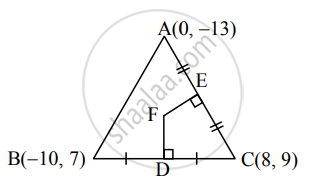
Here, A(0, −13), B(−10, 7), C(8, 9) are the vertices of ΔABC.
Let F be the circumcentre of ΔABC.
Let FD and FE be perpendicular bisectors of the sides BC and AC respectively.
∴ D and E are the midpoints of side BC and AC.
∴ D ≡ `((-10 + 8)/2, (7 + 9)/2)`
∴ D = (−1, 8)
and E ≡ `((0 + 8)/2, (-13 + 9)/2)`
∴ E = (4, −2)
Now, slope of BC = `(7 - 9)/(-10 - 8) = 1/9`
∴ Slope of FD = − 9 ...[∵ FD ⊥ BC]
Since FD passes through (−1, 8) and has slope −9, equation of FD is
y − 8 = −9(x + 1)
∴ y − 8 = −9x − 9
∴ y = −9x − 1 ...(i)
Also, slope of AC = `(-13 - 9)/(0 - 8) = 11/4`
∴ Slope of FE = `(-4)/11` ...[∵ FE ⊥ AC]
Since FE passes through (4, −2) and has slope `(-4)/11`, equation of FE is
y + 2 = `(-4)/11(x - 4)`
∴ 11(y + 2) = −4(x − 4)
∴ 11y + 22 = −4x + 16
∴ 4x + 11y = − 6 ...(ii)
To find coordinates of circumcentre, we have to solve equations (i) and (ii).
Substituting the value of y in (ii), we get
4x + 11(−9x − 1) = − 6
∴ 4x − 99x − 11 = − 6
∴ −95x = 5
∴ x = `(-1)/19`
Substituting the value of x in (i), we get
y = `-9((-1)/19) - 1 = (-10)/19`
∴ Co-ordinates of circumcentre F ≡ `((-1)/19, (-10)/19)`.
APPEARS IN
संबंधित प्रश्न
Find the slope, X-intercept, Y-intercept of the following line:
2x + 3y – 6 = 0
Write the following equation in ax + by + c = 0 form.
y = 2x – 4
Write the following equation in ax + by + c = 0 form.
y = 4
Write the following equation in ax + by + c = 0 form.
`x/2 + y/4` = 1
Show that lines x – 2y – 7 = 0 and 2x − 4y + 15 = 0 are parallel to each other
If the line 3x + 4y = p makes a triangle of area 24 square unit with the co-ordinate axes then find the value of p.
Find the distance of the origin from the line 7x + 24y – 50 = 0
Find the distance between parallel lines 4x − 3y + 5 = 0 and 4x − 3y + 7 = 0
Find points on the line x + y − 4 = 0 which are at one unit distance from the line 4x + 3y – 10 = 0.
Find the equation of the line parallel to the X-axis and passing through the point of intersection of lines x + y − 2 = 0 and 4x + 3y = 10
If A(4, 3), B(0, 0), and C(2, 3) are the vertices of ∆ABC then find the equation of bisector of angle BAC.
D(−1, 8), E(4, −2), F(−5, −3) are midpoints of sides BC, CA and AB of ∆ABC Find equations of sides of ∆ABC
Select the correct option from the given alternatives:
If A(1, −2), B(−2, 3) and C(2, −5) are the vertices of ∆ABC, then the equation of the median BE is
Select the correct option from the given alternatives:
The equation of a line, having inclination 120° with positive direction of X−axis, which is at a distance of 3 units from the origin is
Select the correct option from the given alternatives:
Distance between the two parallel lines y = 2x + 7 and y = 2x + 5 is
Answer the following question:
Find the distance of the origin from the line x = – 2
Answer the following question:
Which of the following lines passes through the origin?
Answer the following question:
Obtain the equation of the line which is parallel to the Y−axis and 2 units to the left of it.
Answer the following question:
Obtain the equation of the line which is parallel to the X−axis and making an intercept of 5 on the Y−axis.
Answer the following question:
Obtain the equation of the line which is parallel to the Y−axis and making an intercept of 3 on the X−axis.
Answer the following question:
Find the distance of the origin from the line 12x + 5y + 78 = 0
Answer the following question:
Find the equation of the line which passes through the point of intersection of lines x + y − 3 = 0, 2x − y + 1 = 0 and which is parallel X-axis
Answer the following question:
Find the equation of the line which passes through the point of intersection of lines x + y + 9 = 0, 2x + 3y + 1 = 0 and which makes X-intercept 1.
Answer the following question:
Find the distance of the line 4x − y = 0 from the point P(4, 1) measured along the line making an angle of 135° with the positive X-axis
A particle is moving in a straight line according to as S = 24t + 3t2 - t3, then the time it will come to rest is ______
For the lines 5x + 2y = 8 and 5x - 2y = 7, which of the following statement is true?
The length of perpendicular from (1, 3) on line 3x + 4y + 10 = 0, is ______
The y-intercept of the line passing through A( 6, 1) and perpendicular to the line x - 2y = 4 is ______.
The equation 12x2 + 7xy + ay2 + 13x - y + 3 = 0 represents a pair of perpendicular lines. Then the value of 'a' is ______
If a plane has x-intercept l, y-intercept m and z-intercept n, and perpendicular distance of plane from the origin is k, then _______.
The length of the perpendicular from the origin on the line `(xsinalpha)/"b" - (ycosalpha)/"a" - 1 = 0` is ______.
If the distance of the point (1, 1, 1) from the origin is half its distance from the plane x + y + z + k = 0, then k = ______.
